A Square Is Inscribed In An Isosceles Right Triangle Finding The Area And Side Length Of The Square

By viagraultimate On Nov 11, 2024 Last updated

A Square Is Inscribed In A Right Isosceles Triangle Such That Two Of In this educational video, we explore the fascinating concept of inscribing a square within an isosceles right triangle. join us as we delve into the mathema. A square with side length is inscribed in an isosceles triangle with one side of the square along the base of the triangle. a square with side length has two vertices on the other square and the other two on sides of the triangle, as shown. what is the area of the triangle? solution 1. let's split the triangle down the middle and label it: we.

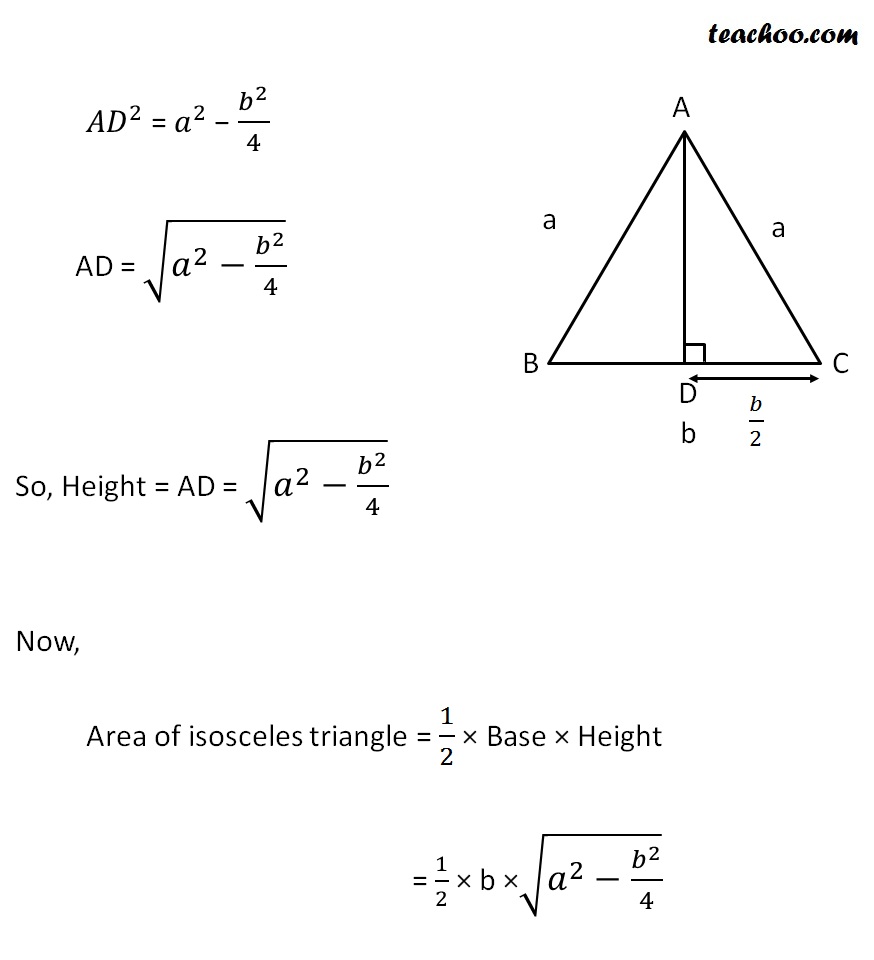
How To Find The Area Of Isosceles Right Triangle At Ruth Caine Blog B. in a right triangle, the square of the length of the hypotenuse equals the difference of the squares of the lengths of the legs. c. if the square of the length c of one side of a triangle equals the sum of the square of the lengths of the legs, then it is a right triangle with the hypotenuse of length c. d. To find the area of an isosceles right triangle, we use the formula: area = ½ × base × height. in this instance, one of the equal sides is considered the base and the other the height. so, if the two equal sides have a measurement of 4cm, insert the measurements for the base and the height to find the actual area. next, follow these steps:. 4. reflect the triangle across its legs ab a b and bc b c. because ab = bc = 2 a b = b c = 2, the resulting figure is a square. moreover, since the inscribed yellow shape is also a square, it is easy to see that their reflections must form a central square that is congruent, and in fact, the entire figure consists of 9 9 congruent squares. Let side of square = s ac = b, bc = a, ab = c. fb = as b and ae = bs a as the colored triangles are similar to the bigger triangle. steps to calculate area (s^2) : 1)calculate gb and ad using right angle triangle rule for triangles gbf and ade. 2)calculate gd using right angle triangle rule for triangle gcd. 3) gd^2 = s^2.

24 Bdef Is A Square Formed Inside An Isosceles Right Triangle Abc 4. reflect the triangle across its legs ab a b and bc b c. because ab = bc = 2 a b = b c = 2, the resulting figure is a square. moreover, since the inscribed yellow shape is also a square, it is easy to see that their reflections must form a central square that is congruent, and in fact, the entire figure consists of 9 9 congruent squares. Let side of square = s ac = b, bc = a, ab = c. fb = as b and ae = bs a as the colored triangles are similar to the bigger triangle. steps to calculate area (s^2) : 1)calculate gb and ad using right angle triangle rule for triangles gbf and ade. 2)calculate gd using right angle triangle rule for triangle gcd. 3) gd^2 = s^2. A square with side length is inscribed in a right triangle with sides of length , , and so that one vertex of the square coincides with the right angle vertex of the triangle. a square with side length is inscribed in another right triangle with sides of length , , and so that one side of the square lies on the hypotenuse of the triangle. what. Find the area. solution: for an isosceles right triangle, the area formula is given by x 2 2 where x is the length of the congruent sides. here, x = 8 units. thus, area = 8 2 2 = 32 square units. therefore, the required area is 32 square units. example 2: the perimeter of an isosceles right triangle is 10 5√2.

Whether you're looking for practical how-to guides, in-depth analyses, or thought-provoking discussions, we are has got you covered. Our diverse range of topics ensures that there's something for everyone, from A Square Is Inscribed In An Isosceles Right Triangle Finding The Area And Side Length Of The Square. We're committed to providing you with valuable information that resonates with your interests.

A square is inscribed in an isosceles right triangle; Finding the area and side length of the square

A square is inscribed in an isosceles right triangle; Finding the area and side length of the square finding the side length of a square inscribed in a right triangle Find the side length of an inscribed square in a 'Right Triangle' | Similar triangles || Similarity Geometry! What is the area above a circle inscribed in an isosceles triangle?? How To Find The Area of an Isosceles Triangle - Math A Rectangle is Inscribed in an Isosceles Triangle - Maximize the Area! Square inside a right triangle; the maximum possible of a square inscribed in a right triangle #math Find the side length of equilateral triangle inscribed in a square How to find the area of intersection between two circles using double integration Find the Area of an Isosceles Right Triangle Find the Area of a Square Located Inside a Right Triangle - 4 Methods Three square on an isosceles right triangle Square inside right triangle problem | Geometry | Advanced math problems | Mathematics A square is inscribed in an isosceles right triangle so that the square and the triangle have one a Square Inscribed in a Circle A triangle with an inscribed square, find the area of the shaded region! Isosceles triangle is inscribed in a square in two ways. A square inside an isosceles triangle #math Geometry : Area of square inscribed in a triangle | GRE, GMAT, CAT, SAT, ACT Find square value of a side of an isosceles triangle Olympiad Mathematics | Find area of the pink square | (Isosceles triangles) | #math #maths

Conclusion

Considering all the aspects, it is unmistakable that content provides useful awareness regarding A Square Is Inscribed In An Isosceles Right Triangle Finding The Area And Side Length Of The Square. All the way through, the blogger reveals remarkable understanding about the area of interest. Crucially, the review of Y stands out as extremely valuable. Additionally, the document shines in disentangling complex concepts in an simple manner. Moreover, the writer shares applicable examples that improve the relatability. An extra component that distinguishes this content is the profound exploration of varied aspects related to A Square Is Inscribed In An Isosceles Right Triangle Finding The Area And Side Length Of The Square. The content creators meticulous approach validates that viewers receive a holistic view of the subject matter. Thank you for your attention to this article. If you have any questions, please feel free to contact me through the medium of social media. I am interested in your messages. In finishing up, for further insights, highlighted below are several pertinent articles that could potentially be enlightening:Happy learning!