Area Equation Of Equilateral Triangle
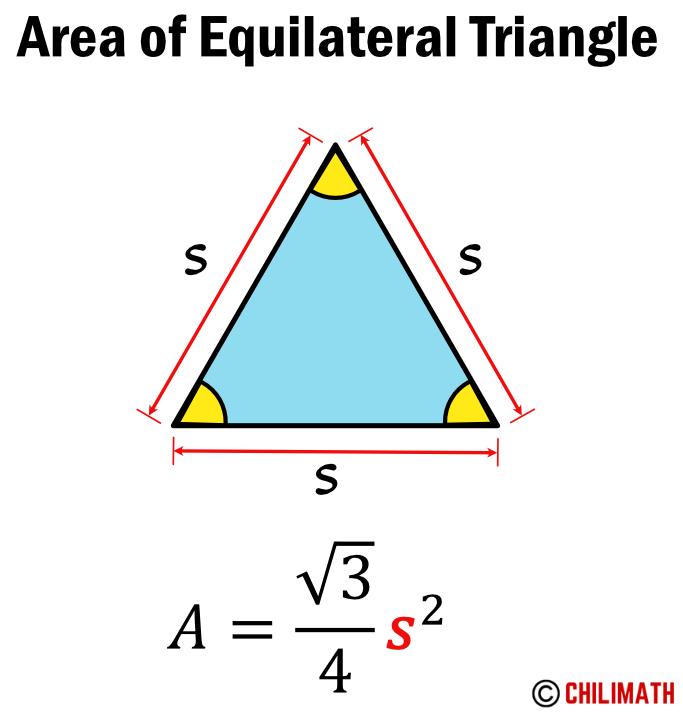
Area Of Equilateral Triangle Derivation Formula Examples Chilimath Let’s plug in the value of the area then solve for the side: learn the method for calculating the area of an equilateral triangle using the formula area = (√3 4)s^2, where 's' denotes the side length of the equilateral triangle. keep in mind that all sides of an equilateral triangle are of equal length. Examples on area of equilateral triangle. example 1: find the area of an equilateral triangle of side 9 cm. solution: the formula for the area of an equilateral triangle is given as, area = √ (3) 4 × (side) 2. by substituting the value of side length in the above formula, we get, = √ (3) 4 × 9 2. = 35.07 inches 2.

Area Of An Equilateral Triangle Formula Examples Definition As per formula: perimeter of the equilateral triangle = 3a, where “a” is the side of the equilateral triangle. step 1: find the side of an equilateral triangle using perimeter. 3a = 12. a = 4. thus, the length of side is 4 cm. step 2: find the area of an equilateral triangle using formula. area, a = √3 a 2 4 sq units. H = a × √3 2. substituting h into the first area formula, we obtain the equation for the equilateral triangle area: area = a² × √3 4. 2. using trigonometry. let's start with the trigonometric triangle area formula: area = (1 2) × a × b × sin(γ), where γ is the angle between the sides. we remember that all sides and all angles. The area of an equilateral triangle is the amount of the space inside an equilateral triangle. an equilateral triangle is a triangle where all angles and sides are equal – making it a regular polygon. since the angles in a triangle add up to 180∘, 180∘, each of the interior angles (internal angles) in an equilateral triangle is 60∘. 60∘. Area of an equilateral triangle $= \frac{\sqrt{3}}{4}\times(a)^2 = \frac{\sqrt{3}}{4}\times(4)^2 = 4\sqrt{3}$ square units. derivation of area of an equilateral triangle formula. all the sides are equal and all the internal angles are 60° in an equilateral triangle. the formula to calculate the area of an equilateral triangle is given as,.

Area Of An Equilateral Triangle Formula Examples Definition The area of an equilateral triangle is the amount of the space inside an equilateral triangle. an equilateral triangle is a triangle where all angles and sides are equal – making it a regular polygon. since the angles in a triangle add up to 180∘, 180∘, each of the interior angles (internal angles) in an equilateral triangle is 60∘. 60∘. Area of an equilateral triangle $= \frac{\sqrt{3}}{4}\times(a)^2 = \frac{\sqrt{3}}{4}\times(4)^2 = 4\sqrt{3}$ square units. derivation of area of an equilateral triangle formula. all the sides are equal and all the internal angles are 60° in an equilateral triangle. the formula to calculate the area of an equilateral triangle is given as,. 2. =. 187.34. when you know all three sides of a triangle, you can find the area using heron's formula. but in the case of equilateral triangles, where all three sides are the same length, there is a simpler formula: area. =. √. Solution: area of equilateral triangle = √3a 2 4, where a is the side. given, a = 20 inches. therefore, area = √3×20×20 4. area of an equilateral triangle = 100√3 square inches. example 2: what is the perimeter of an equilateral triangle whose sides are 40 inches. also, find the height of an equilateral triangle.

Comments are closed.