Calculus 2 Hyperbolic Functions 28 Of 57 Integral Of Hyperbolic

Calculus 2 Hyperbolic Functions 28 Of 57 Integral Of Hyperbolic Visit ilectureonline for more math and science lectures!in this video i will find the integral of [csch^2(x^1 2)] (x^1 2)dx=?next video in the ser. The other hyperbolic functions are then defined in terms of \(\sinh x\) and \(\cosh x\). the graphs of the hyperbolic functions are shown in figure \(\pageindex{1}\). figure \(\pageindex{1}\): graphs of the hyperbolic functions. it is easy to develop differentiation formulas for the hyperbolic functions. for example, looking at \(\sinh x\) we have.
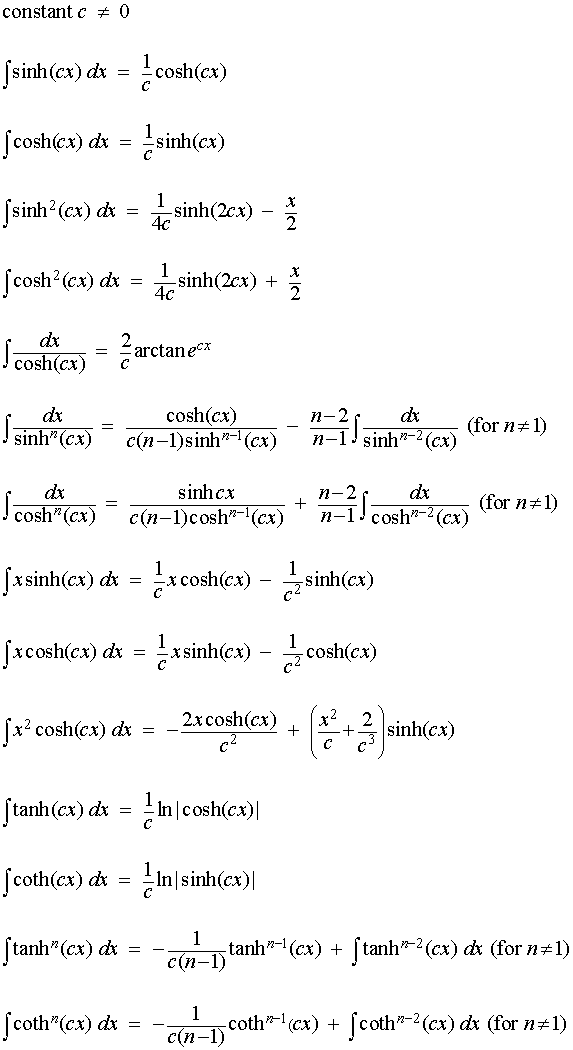
List Of Integrals Of Hyperbolic Functions 6.9.1 apply the formulas for derivatives and integrals of the hyperbolic functions. 6.9.2 apply the formulas for the derivatives of the inverse hyperbolic functions and their associated integrals. 6.9.3 describe the common applied conditions of a catenary curve. The hyperbolic functions have identities that are similar to those of trigonometric functions: since the hyperbolic functions are expressed in terms of and we can easily derive rules for their differentiation and integration: in certain cases, the integrals of hyperbolic functions can be evaluated using the substitution. To retrieve these formulas we rewrite the de nition of the hyperbolic function as a degree two polynomial in ex; then we solve for ex and invert the exponential. for example: ex e x. y = sinh x = , e2x 2yex 1 = 0 , ex = y py2 1. 2. and since the exponential must be positive we select the positive sign. Hyperbolic functions are defined in terms of exponential functions. term by term differentiation yields differentiation formulas for the hyperbolic functions. these differentiation formulas give rise, in turn, to integration formulas. with appropriate range restrictions, the hyperbolic functions all have inverses.
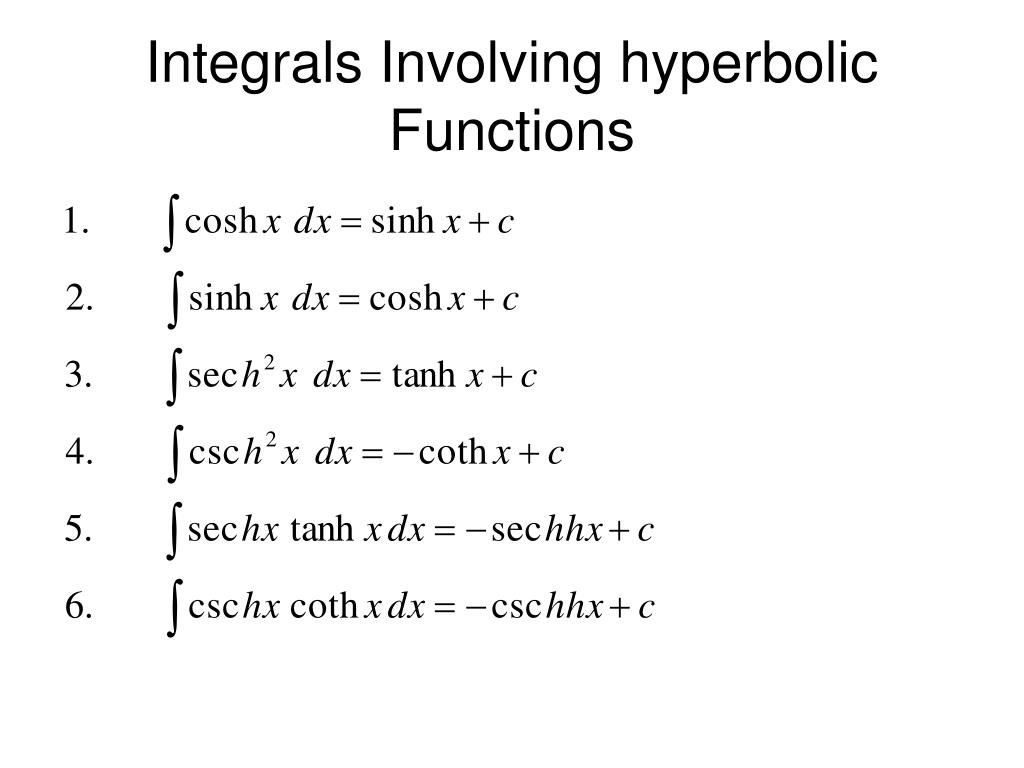
Ppt Hyperbolic Functions Powerpoint Presentation Free Download Id To retrieve these formulas we rewrite the de nition of the hyperbolic function as a degree two polynomial in ex; then we solve for ex and invert the exponential. for example: ex e x. y = sinh x = , e2x 2yex 1 = 0 , ex = y py2 1. 2. and since the exponential must be positive we select the positive sign. Hyperbolic functions are defined in terms of exponential functions. term by term differentiation yields differentiation formulas for the hyperbolic functions. these differentiation formulas give rise, in turn, to integration formulas. with appropriate range restrictions, the hyperbolic functions all have inverses. 2.1 definitions. the hyperbolic cosine function, written cosh x, is defined for all real values of x by the relation. 1. cosh x = ex x. e. 2 ( ) ion, sinh x, is defined by1sinh x =(ex e x2 )the names of these two hyperbolic functions suggest that they have similar properties to the trigonome. ctivity 1show t. What you’ll learn to do: use integrals and derivatives to evaluate hyperbolic functions. we were introduced to hyperbolic functions in module 1: functions and graphs, along with some of their basic properties. in this section, we look at differentiation and integration formulas for the hyperbolic functions and their inverses.

Ppt Hyperbolic Functions Powerpoint Presentation Id 4004536 2.1 definitions. the hyperbolic cosine function, written cosh x, is defined for all real values of x by the relation. 1. cosh x = ex x. e. 2 ( ) ion, sinh x, is defined by1sinh x =(ex e x2 )the names of these two hyperbolic functions suggest that they have similar properties to the trigonome. ctivity 1show t. What you’ll learn to do: use integrals and derivatives to evaluate hyperbolic functions. we were introduced to hyperbolic functions in module 1: functions and graphs, along with some of their basic properties. in this section, we look at differentiation and integration formulas for the hyperbolic functions and their inverses.

Calculus 2 Hyperbolic Functions 30 Of 57 Why Do We Need Inverse

Comments are closed.