Classification Of Second Order Partial Differential Equation

Partial Differential Equation Of Second Order Youtube This is known as the classification of second order pdes. let u = u(x, y). then, the general form of a linear second order partial differential equation is given by. a(x, y)uxx 2b(x, y)uxy c(x, y)uyy d(x, y)ux e(x, y)uy f(x, y)u = g(x, y). in this section we will show that this equation can be transformed into one of three types of. Dy b √b2 4ac. = − − (7.7) dx 2a. along these directions the partial differential equation takes a simple form called nor mal or canonical form. further, the curves (7.6) and (7.7) are called characteristic curves. consider the hyperbolic equation (7.5), for which the characteristic curves can be obtained using. dx √4a2.
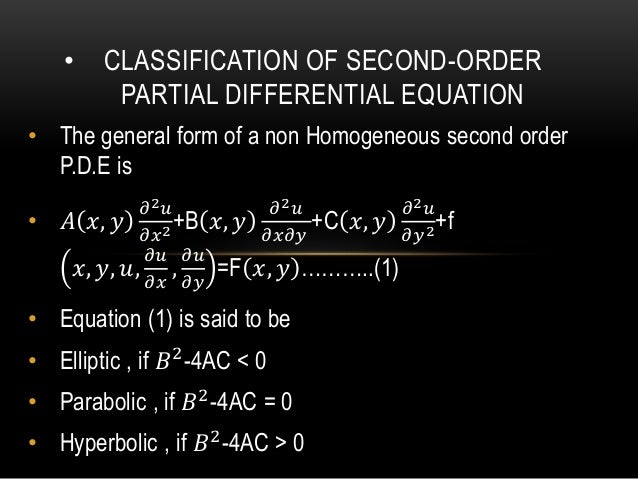
Classification Of Second Order Partial Differential Equation The classification of second order partial differential equations (3.1), is suggested by the classification of the above equation (2), and based upon the possibilities of transforming equation (1) to canonical form at any point (x 0, y 0) by suitable coordinate transformation. therefore, an equation at any point (x 0, y 0) is called. Partial differential equations occur in many different areas of physics, chemistry and engineering. 2.2: second order pde. second order p.d.e. are usually divided into three types: elliptical, hyperbolic, and parabolic. 2.3: more than 2d. in more than two dimensions we use a similar definition, based on the fact that all eigenvalues of the. Microsoft word 203.lecture.5. 5. classification of second order equations. there are 2 general methods for classifying higher order partial differential equations. one is very general (applying even to some nonlinear equations), and seems to have been motivated by the success of the theory of first order pdes. Introduction. in this chapter, we discuss the classification of partial differentialequations (pde), concentrating mostly on linear equations or equations withlinear principal part. in the modern theory of the subject, especially sincethe middle of the last century, the question of classification has takenaltogether new directions, which we.

Classification Of Partial Differential Equations Of Second Order Microsoft word 203.lecture.5. 5. classification of second order equations. there are 2 general methods for classifying higher order partial differential equations. one is very general (applying even to some nonlinear equations), and seems to have been motivated by the success of the theory of first order pdes. Introduction. in this chapter, we discuss the classification of partial differentialequations (pde), concentrating mostly on linear equations or equations withlinear principal part. in the modern theory of the subject, especially sincethe middle of the last century, the question of classification has takenaltogether new directions, which we. Second order partial differential equations 3 of harmony. this idea was carried further by johannes kepler (1571 1630) in his harmony of the spheres approach to planetary orbits. in the 1700’s oth ers worked on the superposition theory for vibrating waves on a stretched spring, starting with the wave equation and leading to the superposition. Second order partial differential equations not unique. there are also other kinds of boundary conditions. for example, we may specify the value of u at one of the boundary points, and the value of u at the other boundary point. as an example, let u 0 1 and u 1 0. then the solution of the boundary value problem exists and is unique, and.

Comments are closed.