Classifications Of Second Order Partial Differential Equations

Classifications Of Second Order Partial Differential Equations This is known as the classification of second order pdes. let u = u(x, y). then, the general form of a linear second order partial differential equation is given by. a(x, y)uxx 2b(x, y)uxy c(x, y)uyy d(x, y)ux e(x, y)uy f(x, y)u = g(x, y). in this section we will show that this equation can be transformed into one of three types of. Partial differential equations occur in many different areas of physics, chemistry and engineering. 2.2: second order pde. second order p.d.e. are usually divided into three types: elliptical, hyperbolic, and parabolic. 2.3: more than 2d. in more than two dimensions we use a similar definition, based on the fact that all eigenvalues of the.

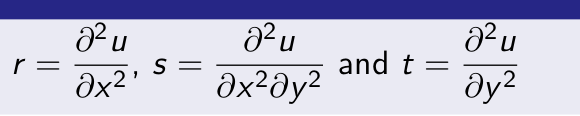
Classification Of Second Order Partial Differential Equations Dy b √b2 4ac. = − − (7.7) dx 2a. along these directions the partial differential equation takes a simple form called nor mal or canonical form. further, the curves (7.6) and (7.7) are called characteristic curves. consider the hyperbolic equation (7.5), for which the characteristic curves can be obtained using. dx √4a2. Microsoft word 203.lecture.5. 5. classification of second order equations. there are 2 general methods for classifying higher order partial differential equations. one is very general (applying even to some nonlinear equations), and seems to have been motivated by the success of the theory of first order pdes. That is, there are several independent variables. 🔗. 🔗. let us see some examples of ordinary differential equations: (exponential growth) (newton's law of cooling) (mechanical vibrations) d y d t = k y, (exponential growth) d y d t = k (a − y), (newton's law of cooling) m d 2 x d t 2 c d x d t k x = f (t). (mechanical vibrations) 🔗. Introduction. in this chapter, we discuss the classification of partial differentialequations (pde), concentrating mostly on linear equations or equations withlinear principal part. in the modern theory of the subject, especially sincethe middle of the last century, the question of classification has takenaltogether new directions, which we.

Classification Of Second Order P D E Partial Differential Equation That is, there are several independent variables. 🔗. 🔗. let us see some examples of ordinary differential equations: (exponential growth) (newton's law of cooling) (mechanical vibrations) d y d t = k y, (exponential growth) d y d t = k (a − y), (newton's law of cooling) m d 2 x d t 2 c d x d t k x = f (t). (mechanical vibrations) 🔗. Introduction. in this chapter, we discuss the classification of partial differentialequations (pde), concentrating mostly on linear equations or equations withlinear principal part. in the modern theory of the subject, especially sincethe middle of the last century, the question of classification has takenaltogether new directions, which we. Math 124a partial differential equations paul j. atzberger the second order terms turn out to dominate the behavior of the pde, so we will write the differential operator asl= l 2 l 1 l 0, where l 2 contains the second order terms and l 1 the first order terms, andl 0 the constant. let l 2[u] = a 11u x 1x 1 2a 12u x 1x 2 a 22u x 2x 2 l 1. The study of boundary value problems for ordinary differential equations. these generic differential equation occur in one to three spatial dimensions and are all linear differential equations. a list is provided in table 1.1. here we have introduced the laplacian operator, r2u = uxx uyy uzz. depend ing on the types of boundary conditions.

Table 1 1 From Classification Of Second Order Partial Differential Math 124a partial differential equations paul j. atzberger the second order terms turn out to dominate the behavior of the pde, so we will write the differential operator asl= l 2 l 1 l 0, where l 2 contains the second order terms and l 1 the first order terms, andl 0 the constant. let l 2[u] = a 11u x 1x 1 2a 12u x 1x 2 a 22u x 2x 2 l 1. The study of boundary value problems for ordinary differential equations. these generic differential equation occur in one to three spatial dimensions and are all linear differential equations. a list is provided in table 1.1. here we have introduced the laplacian operator, r2u = uxx uyy uzz. depend ing on the types of boundary conditions.

09 Classification Of Second Order Partial Differential Equations