Definition Statistics And Probability Concepts Multiplication Rule Of

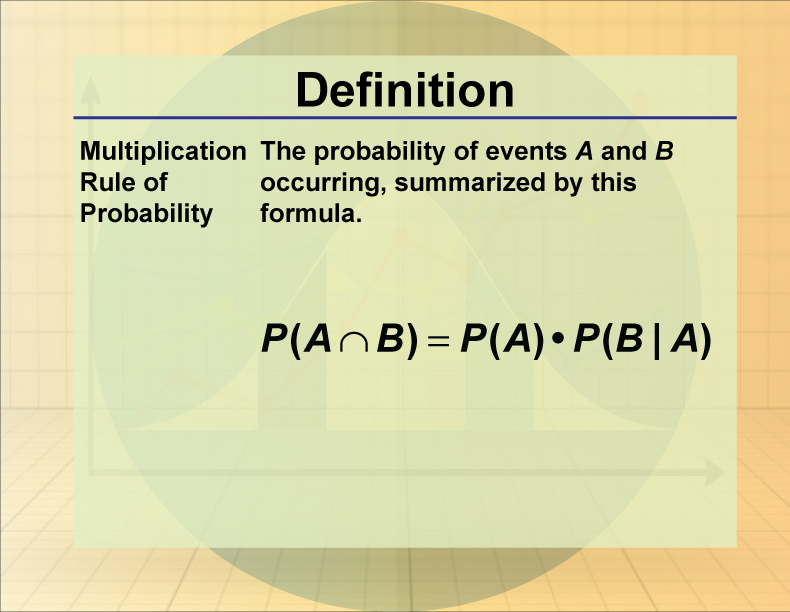
Definition Statistics And Probability Concepts Multiplication Rule Of The addition rule. if a and b are defined on a sample space, then: p (a or b) = p (a) p (b) − p (a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.3.2 becomes. p (a or b) = p (a) p (b). example 4.3.1. klaus is trying to choose where to go on vacation. We have already learned the multiplication rules we follow in probability, such as; p (a∩b) = p (a)×p (b|a) ; if p (a) ≠ 0. p (a∩b) = p (b)×p (a|b) ; if p (b) ≠ 0. let us learn here the multiplication theorems for independent events a and b. if a and b are two independent events for a random experiment, then the probability of.

Ppt Chapter 15 Probability Rules Powerpoint Presentation Free Multiplication rule probability: using the specific rule. using the specific multiplication rule formula is very straightforward. just multiply the probability of the first event by the second. for example, if the probability of event a is 2 9 and the probability of event b is 3 9 then the probability of both events happening at the same time. The multiplication rule is a fundamental principle in probability that helps determine the likelihood of multiple events occurring together. it connects individual event probabilities to find the combined probability of two or more events happening simultaneously. this rule is essential for understanding how independent and dependent events interact, linking it closely to axioms of probability. The general multiplication rule states that the probability of any two events, a and b, both happening can be calculated as: p (a and b) = p (a) * p (b|a) the vertical bar | means “given.”. thus, p (b|a) can be read as “the probability that b occurs, given that a has occurred.”. if events a and b are independent, then p (b|a) is simply. Example 4.4.1 4.4. 1. klaus is trying to choose where to go on vacation. his two choices are: a = new zealand a = new zealand and b = alaska b = alaska. klaus can only afford one vacation. the probability that he chooses a a is p(a) = 0.6 p (a) = 0.6 and the probability that he chooses b b is p(b) = 0.35 p (b) = 0.35.

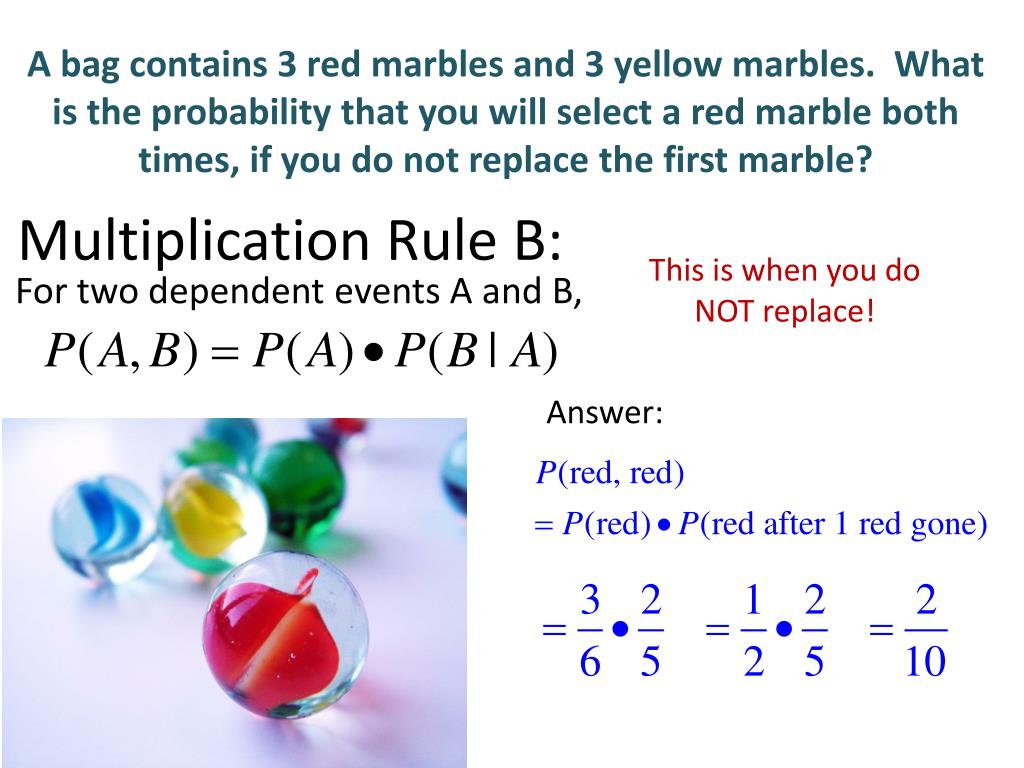
Ppt Unit 4 Probability Simple Probability Examples Powerpoint The general multiplication rule states that the probability of any two events, a and b, both happening can be calculated as: p (a and b) = p (a) * p (b|a) the vertical bar | means “given.”. thus, p (b|a) can be read as “the probability that b occurs, given that a has occurred.”. if events a and b are independent, then p (b|a) is simply. Example 4.4.1 4.4. 1. klaus is trying to choose where to go on vacation. his two choices are: a = new zealand a = new zealand and b = alaska b = alaska. klaus can only afford one vacation. the probability that he chooses a a is p(a) = 0.6 p (a) = 0.6 and the probability that he chooses b b is p(b) = 0.35 p (b) = 0.35. To use this rule, multiply the probabilities for the independent events. with independent events, the occurrence of event a does not affect the likelihood of event b. this rule is not valid for dependent events. using probability notation, the specific multiplication rule is the following: p (a ∩ b) = p (a) * p (b) or, the joint probability. The multiplication rule is a fundamental principle in probability that provides a way to calculate the probability of the intersection of two or more events. this rule connects directly to conditional probability, where the occurrence of one event affects the probability of another event. understanding this rule is crucial for determining probabilities in scenarios where events are dependent.

Multiplication Rule Of Probability Probability Theory Intersection To use this rule, multiply the probabilities for the independent events. with independent events, the occurrence of event a does not affect the likelihood of event b. this rule is not valid for dependent events. using probability notation, the specific multiplication rule is the following: p (a ∩ b) = p (a) * p (b) or, the joint probability. The multiplication rule is a fundamental principle in probability that provides a way to calculate the probability of the intersection of two or more events. this rule connects directly to conditional probability, where the occurrence of one event affects the probability of another event. understanding this rule is crucial for determining probabilities in scenarios where events are dependent.

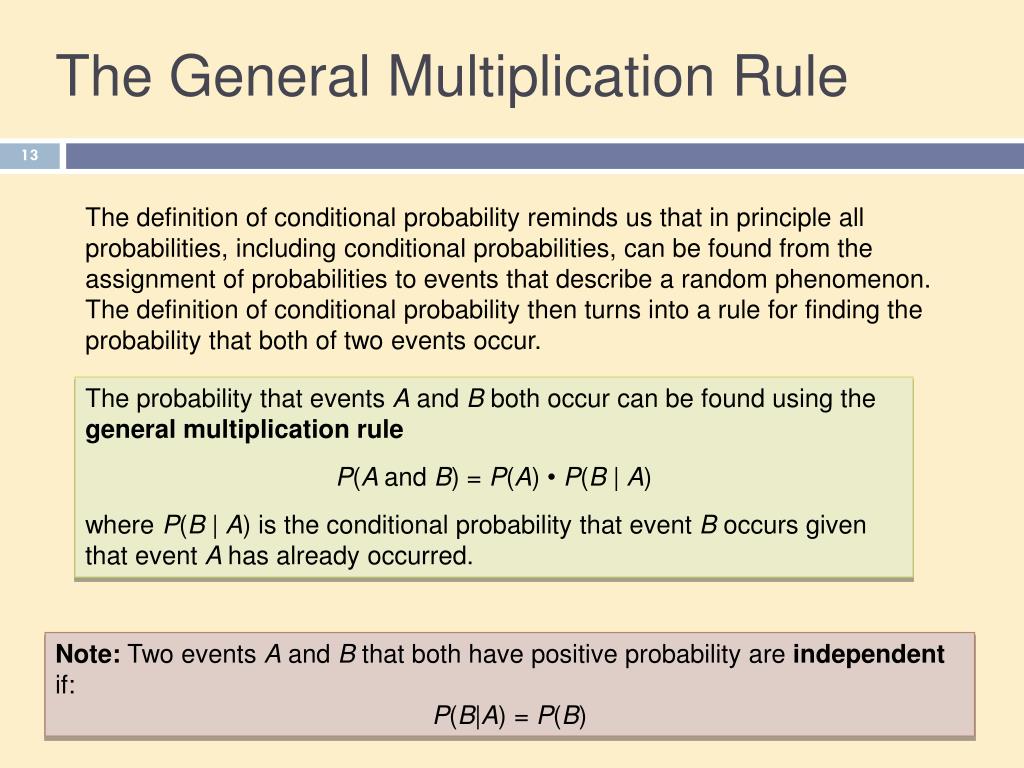
Ppt Chapter 12 General Rules Of Probability Powerpoint Presentation

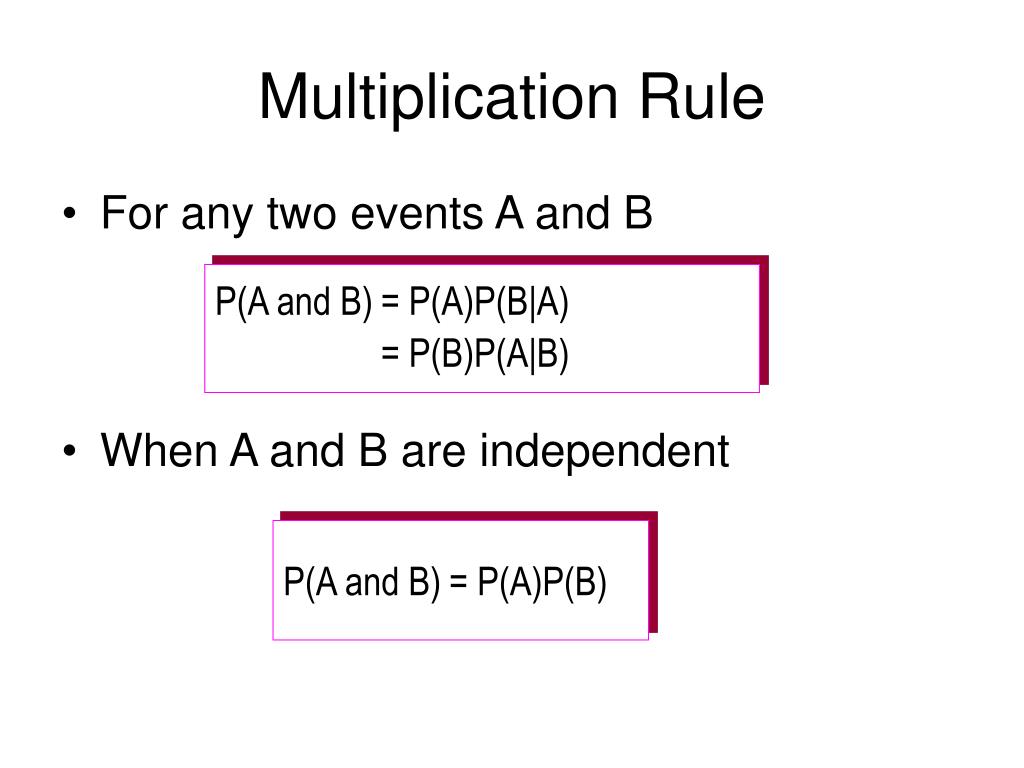
Ppt Chapter 6 Probability Powerpoint Presentation Free Download Id