Dividing Polynomials And The Remainder Theorem Solutions Examples

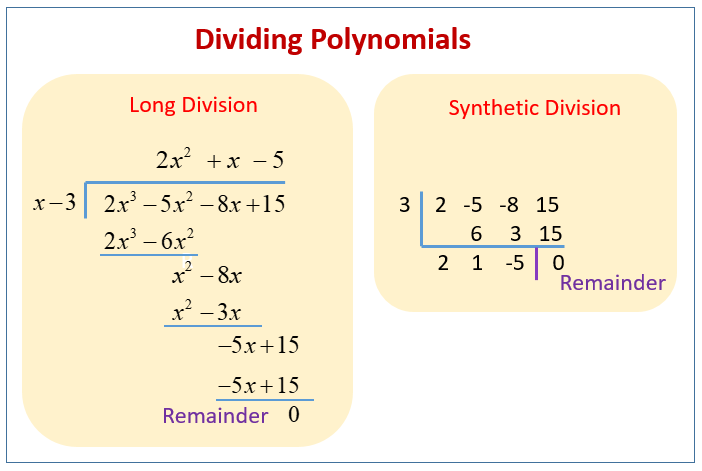
Dividing Polynomials And The Remainder Theorem Solutions Examples According to this theorem, if we divide a polynomial p(x) by a factor ( x – a); that isn’t essentially an element of the polynomial; you will find a smaller polynomial along with a remainder. this remainder that has been obtained is actually a value of p(x) at x = a, specifically p(a) . F (c) = r. so we get this: the remainder theorem: when we divide a polynomial f (x) by x−c the remainder is f (c) so to find the remainder after dividing by x c we don't need to do any division: just calculate f (c) let us see that in practice: example: the remainder after 2x 2 −5x−1 is divided by x−3.

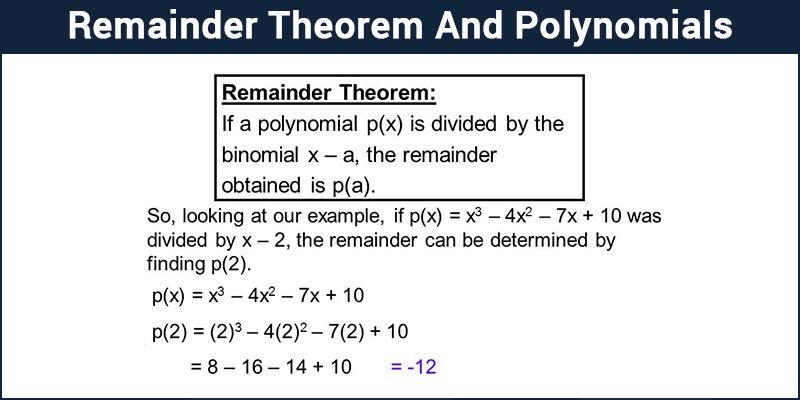
Remainder Theorem Remainder Theorem Of Polynomial Examples Remember, we started with a third degree polynomial and divided by a first degree polynomial, so the quotient is a second degree polynomial. hence the quotient is \(x^{2} 6 x 7\). the number in the box is the remainder. synthetic division is our tool of choice for dividing polynomials by divisors of the form \(x − c\). Examples, solutions, videos, worksheets, and activities to help algebra and grade 9 students learn about dividing polynomials and the remainder theorem. the following diagrams show how to divide polynomials using long division and synthetic division. scroll down the page for more examples and solutions. this lesson shows how to divide a. The remainder theorem states that when a polynomial p(x) is divided by a linear polynomial (x a), then the remainder is equal to p(a). the remainder theorem enables us to calculate the remainder of the division of any polynomial by a linear polynomial, without actually carrying out the steps of the long division. Divide a polynomial by a monomial. now that we know how to divide a monomial by a monomial, the next procedure is to divide a polynomial of two or more terms by a monomial. the method we’ll use to divide a polynomial by a monomial is based on the properties of fraction addition. so we’ll start with an example to review fraction addition.

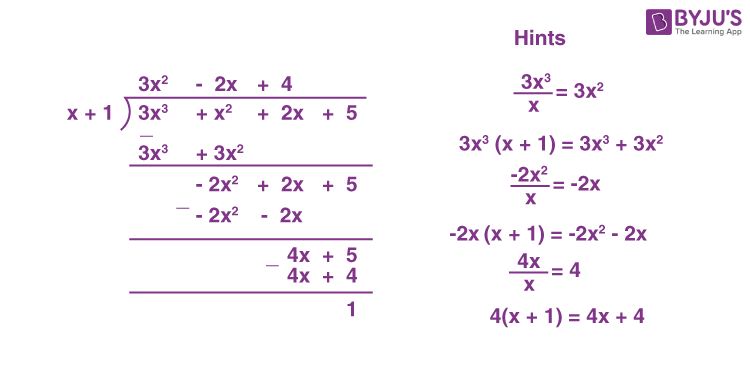
Remainder Theorem Remainder Theorem Of Polynomial Examples The remainder theorem states that when a polynomial p(x) is divided by a linear polynomial (x a), then the remainder is equal to p(a). the remainder theorem enables us to calculate the remainder of the division of any polynomial by a linear polynomial, without actually carrying out the steps of the long division. Divide a polynomial by a monomial. now that we know how to divide a monomial by a monomial, the next procedure is to divide a polynomial of two or more terms by a monomial. the method we’ll use to divide a polynomial by a monomial is based on the properties of fraction addition. so we’ll start with an example to review fraction addition. The remainder theorem. for any polynomial p(x) of degree 1 or higher and any real number k, p(k) is equal to the remainder of the division of p(x) by x − k. algebraically: if p(x) x − k = q(x) r x − k, then p(k) = r. as always, an equality is true “both ways”. in the remainder theorem, we can say that. Based on the theorem, the remainder is always constant (degree 0) as we divide f(x) by a linear polynomial. thus, f(a) = r(a) = r(x) now, let us verify this with an example. here, we will divide the polynomial (3x 5 – 7x 4 – 7x 3 7x 2 – 16x 18) by (x – 3), using three possible methods. using long division.