When Rotating The View It Seems That The Coordinates X And Y Are Question:when rotating the view, it seems that the coordinates x and y are reversed. is there a display problem?answer:the described effect is not a display. Explore math with our beautiful, free online graphing calculator. graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more.
X And Y Coordinates Learn And Solve Questions Exclusive content for member’s only. 00:12:12 – draw the image given the rotation (examples #5 6) 00:16:41 – find the coordinates of the vertices after the given transformation (examples #7 8) 00:19:03 – how to describe the rotation after two repeated reflections (examples #9 10) 00:26:32 – identify rotational symmetry, order, and. 2d rotation about a point. imagine a point located at (x,y). if you wanted to rotate that point around the origin, the coordinates of the new point would be located at (x',y'). x ′ = x cos θ − y sin θ y ′ = y cos θ x sin θ. where θ is the angle of rotation. in matrix notation, this can be written as:. You are rotating the coordinate axes, not the points. let $(x,y)$ be a point in the plane. then $(x,y)=x(1,0) y(0,1)$.a rotation of the coordinates by $45^{\circ. Question #5: a water wheel has a diameter of 20 feet. water from a water trough that is positioned above the water wheel is poured into the paddles of the water wheel to force it to rotate in a clockwise direction. the water in a paddle begins to be released from the water wheel after it makes a 90° rotation.
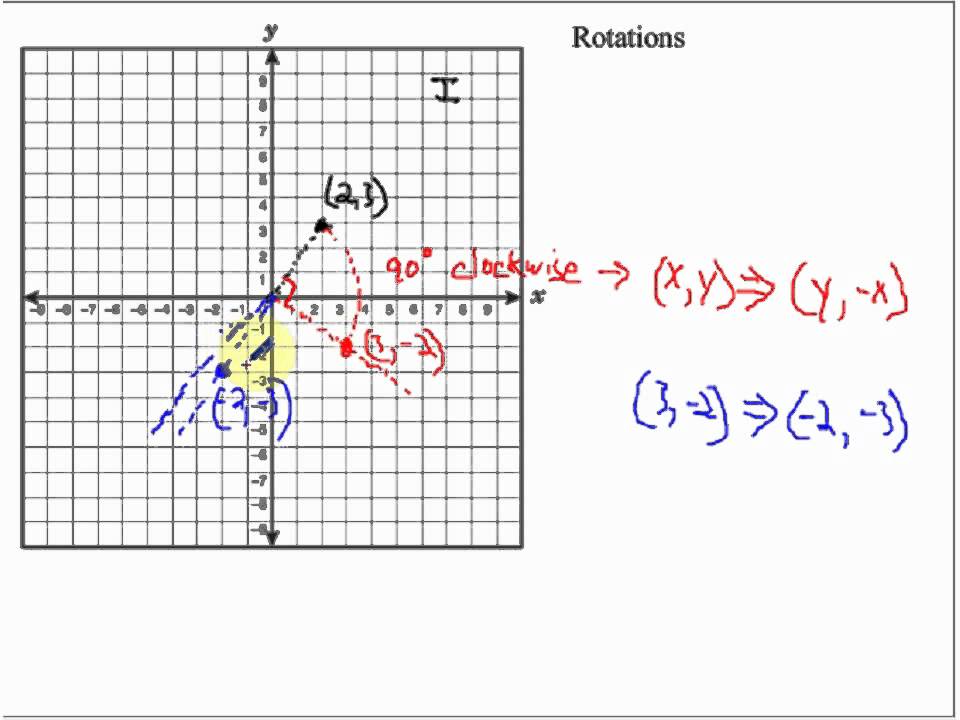
Rotations In The Coordinate Plane Youtube You are rotating the coordinate axes, not the points. let $(x,y)$ be a point in the plane. then $(x,y)=x(1,0) y(0,1)$.a rotation of the coordinates by $45^{\circ. Question #5: a water wheel has a diameter of 20 feet. water from a water trough that is positioned above the water wheel is poured into the paddles of the water wheel to force it to rotate in a clockwise direction. the water in a paddle begins to be released from the water wheel after it makes a 90° rotation. A rotation of − 9 0 ∘ is equivalent to a rotation of 2 7 0 ∘ and therefore results in a point with coordinates (𝑦, − 𝑥). a rotation of − 1 8 0 ∘ is equivalent to a rotation of 1 8 0 ∘ and therefore results in a point with coordinates (− 𝑥, − 𝑦). a rotation of − 2 7 0 ∘ is equivalent to a rotation of 9 0 ∘ and. A rotation is a type of transformation that moves a figure around a central rotation point, called the point of rotation. the point of rotation can be inside or outside of the figure. in this lesson we’ll look at how the rotation of a figure in a coordinate plane determines where it’s located.
Rotations On The Coordinate Plane A rotation of − 9 0 ∘ is equivalent to a rotation of 2 7 0 ∘ and therefore results in a point with coordinates (𝑦, − 𝑥). a rotation of − 1 8 0 ∘ is equivalent to a rotation of 1 8 0 ∘ and therefore results in a point with coordinates (− 𝑥, − 𝑦). a rotation of − 2 7 0 ∘ is equivalent to a rotation of 9 0 ∘ and. A rotation is a type of transformation that moves a figure around a central rotation point, called the point of rotation. the point of rotation can be inside or outside of the figure. in this lesson we’ll look at how the rotation of a figure in a coordinate plane determines where it’s located.
How To Perform Rotations On A Coordinate Plane Geometry Study
Comments are closed.