Equation Of Motion Of Simple Pendulum Using Hamilton S Equation Of

Equation Of Motion Of Simple Pendulum Using Hamilton S Equation Of H = p2 2mr2 mgr(1 − cos θ) h = p 2 2 m r 2 m g r (1 − cos. ⁡. θ) now hamilton's equations will be: p˙ = −mgr sin θ p ˙ = − m g r sin θ. θ˙ = p mr2 θ ˙ = p m r 2. i know one of the points of hamiltonian formalism is to get first order diff. equations instead of second order that lagrangian formalism gives you, but how can. Classical mechanics this video helps to understand the equation of motion derivation using hamilton's equation of motion.

Equation Of Motion Of Simple Pendulum Using Hamilton S Equation This lecture speaks about the derivation of the equation of motion for a simple pendulum using hamiltonian dynamics. the time period is also elucidated at th. University of victoria. in classical mechanics we can describe the state of a system by specifying its lagrangian as a function of the coordinates and their time rates of change: l = l(qi,q˙) (14.3.1) if the coordinates and the velocities increase, the corresponding increment in the lagrangian is. dl = ∑i ∂l ∂qi dqi ∑i ∂l ∂qi. Using this equation, we can find the period of a pendulum for amplitudes less than about \(15^o\). for the simple pendulum: \[t = 2\pi \sqrt{\dfrac{m}{k}} = 2\pi \sqrt{\dfrac{m}{mg l}}.\] for the period of a simple pendulum. this result is interesting because of its simplicity. the only things that affect the period of a simple pendulum are its. 4.1 hamilton’s equations recall that in the lagrangian formulation, we have the function l(q i,q˙ i,t)whereq i (i =1, ,n)aren generalised coordinates. the equations of motion are d dt @l @q˙ i @l @q i =0 (4.1) these are n 2nd order di↵erential equations which require 2n initial conditions, say q i(t =0)and ˙q i(t = 0). the basic idea.

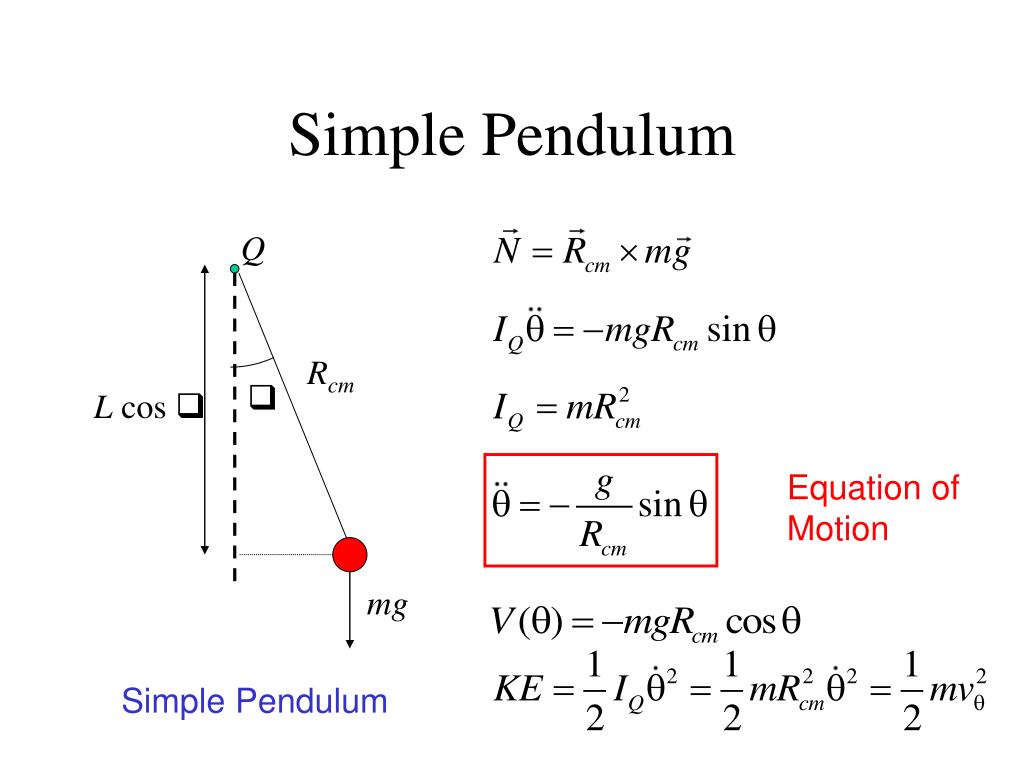
Ppt Simple Pendulum Powerpoint Presentation Free Download Id 814485 Using this equation, we can find the period of a pendulum for amplitudes less than about \(15^o\). for the simple pendulum: \[t = 2\pi \sqrt{\dfrac{m}{k}} = 2\pi \sqrt{\dfrac{m}{mg l}}.\] for the period of a simple pendulum. this result is interesting because of its simplicity. the only things that affect the period of a simple pendulum are its. 4.1 hamilton’s equations recall that in the lagrangian formulation, we have the function l(q i,q˙ i,t)whereq i (i =1, ,n)aren generalised coordinates. the equations of motion are d dt @l @q˙ i @l @q i =0 (4.1) these are n 2nd order di↵erential equations which require 2n initial conditions, say q i(t =0)and ˙q i(t = 0). the basic idea. Hamilton's equations give the time evolution of coordinates and conjugate momenta in four first order differential equations, ˙ = ˙ = ⁡ ˙ = ⁡ ⁡ ⁡ ˙ = momentum ⁠ ⁠, which corresponds to the vertical component of angular momentum ⁠ = ⁡ ⁡ ˙ ⁠, is a constant of motion. that is a consequence of the rotational symmetry of the. The equations of motion in hamiltonian form (6.3), q k= @h @p k q;t; p k= − @h @q k p;t; are almost symmetric in their treatment of q and p. if we de ne a 2n dimensional coordinate for phase space, i = q i n i = p i ˙ for 1 i n; we can write hamilton’s equation in terms of a particular matrix j, j = x2n k=1 j jk @h @ k; where j= 01i n.