Geometry 3 Circles With Radii 66 77 And 88 Externally Tangent To

Geometry 3 Circles With Radii 66 77 And 88 Externally Tangent To The radii of the 3 smaller circles are 66, 77, and 88. what is the radius of the bigger circle? i actually solved this problem by drawing in the center of the bigger circle, drawing lines from that center to the 3 points of tangency on the large circle, then set up a system of equations and go for there (if you want me to be more specific just. 3 circles with radii 66, 77, and 88 externally tangent to each other, find the radius of the circle internally tangent to the other circles. 1 calculating the area between three tangent circles of unit radius, and using the result to show $\pi^2<12$.

Math Principles Three Tangent Circles 3. extend ab a b to e e so that b b between a a and e e and ae = 2. a e = 2. construct a circle of radius 4 4 about a, a, a circle of radius 2 2 about e, e, and a circle of radius 1 1 about c. c. confirm that the circles about a a and e e are internally tangent, the circles about a a and c c are internally tangent, and the circles about c c and. Learn to apply systems of equations in solving geometry problems. how to find the radii of three circles touching each other externally or externally tangent. Created with geogebra. three circles (o), with centers at o, i = 1, 2, 3, touch pairwise externally. if i, j, k are three different indices obtained from 1, 2, 3 by a cyclic permutation, then p k denotes the point of contact of (o) and (o). p p extended crosses again (o) in q k and (o) in r k. the circle (a k) tangent to (o) in q k and (o) in r. Tangent circles. download wolfram notebook. two circles with centers at with radii for are mutually tangent if. (1) if the center of the second circle is inside the first, then the and signs both correspond to internally tangent circles. if the center of the second circle is outside the first, then the sign corresponds to externally tangent.
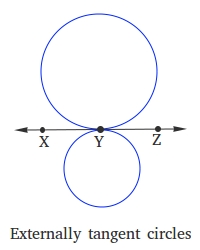
Tangent Circles Created with geogebra. three circles (o), with centers at o, i = 1, 2, 3, touch pairwise externally. if i, j, k are three different indices obtained from 1, 2, 3 by a cyclic permutation, then p k denotes the point of contact of (o) and (o). p p extended crosses again (o) in q k and (o) in r k. the circle (a k) tangent to (o) in q k and (o) in r. Tangent circles. download wolfram notebook. two circles with centers at with radii for are mutually tangent if. (1) if the center of the second circle is inside the first, then the and signs both correspond to internally tangent circles. if the center of the second circle is outside the first, then the sign corresponds to externally tangent. Three circles with radii 1, 2, and 3 ft. are externally tangent to one another, as shown in the figure. find the area of the sector of the circle of radius 1 ft. that is cut off by the line segments joining the center of that circle to the centers of the other two circles. the length of the sides of a triangle is calculated by the sum of the. Circles of radius and are externally tangent to each other and are internally tangent to a circle of radius . the circle of radius has a chord that is a common external tangent of the other two circles. find the square of the length of this chord. solution 1. we label the points as following: the centers of the circles of radii are respectively.

Math Principles Three Tangent Circles Three circles with radii 1, 2, and 3 ft. are externally tangent to one another, as shown in the figure. find the area of the sector of the circle of radius 1 ft. that is cut off by the line segments joining the center of that circle to the centers of the other two circles. the length of the sides of a triangle is calculated by the sum of the. Circles of radius and are externally tangent to each other and are internally tangent to a circle of radius . the circle of radius has a chord that is a common external tangent of the other two circles. find the square of the length of this chord. solution 1. we label the points as following: the centers of the circles of radii are respectively.

Comments are closed.