Geometry What Is The Area Of The Square Inscribed In A 3 4 5 Triangle

Geometry What Is The Area Of The Square Inscribed In A 3 4 5 Triangle We find the area of a square inscribed in a 3 4 5 triangle. this is a pretty straightforward solution, and if you've taken a geometry class, you have all of. Proof of the 3 4 5 triangle. let’s take a look at the math that proves the existence of the 3 4 5 ratio. we are going to use the standard side lengths of 3 and 4 to look for the 3rd side length using the pythagorean theorem. remember that a 2 b 2 = c 2. 3 2 4 2 = 9 16 = 25; 5 2 = 25, so the 3 4 5 right triangle ratio is satisfied.

Can You Calculate The Area Of The Square That Is Inscribed A 3 4 5 What is the area of the square? thanks to papa in india for the suggestion!this puzzle is half of of problem 21 in the 2017 amc 10a. artofproblemsolvi. In a 3 4 5 triangle, a 2 b 2 = c 2, here a = 3 units, b = 4 units. ⇒ 3 2 4 2 = c 2. ⇒ 25 = c 2. ⇒ c = 5 units. hence, it works. other pythagorean triples. 3 4 5 triangle does not mean that the ratios are always exactly 3: 4: 5. but, it can be any factor of numbers, keeping the basic ratio of the three sides the same. Because abc is a 3 4 5 triangle, it is a right triangle. consequently, triangles abc, pbs, src, and aqp are similar triangles. in each triangle, the longer leg to the shorter leg has a ratio of 4 3 (and the shorter leg to longer leg is a ratio of 3 4). suppose the square has a side length equal to s. then qr = s. Learn how to calculate the area of a square that is inside of a three four five (3 4 5) right triangle by using the similarity as well as angle angle angle t.

Area Of Square Inscribed In A Triangle Youtube Because abc is a 3 4 5 triangle, it is a right triangle. consequently, triangles abc, pbs, src, and aqp are similar triangles. in each triangle, the longer leg to the shorter leg has a ratio of 4 3 (and the shorter leg to longer leg is a ratio of 3 4). suppose the square has a side length equal to s. then qr = s. Learn how to calculate the area of a square that is inside of a three four five (3 4 5) right triangle by using the similarity as well as angle angle angle t. Solution 2. the hypotenuse of the triangle is a diameter of the circumcircle, so it has length . the triangle is similar to a 3 4 5 triangle with the ratio of their side lengths equal to . the area of a 3 4 5 triangle is . the square of the ratio of their side lengths is equal to the ratio of their areas. call the area of the triangle . The 3,4,5 triangle has a simple beauty. at its heart is a circle: the circle is a unit circle: it has a radius of exactly 1 and an area of π. and using that circle we can cut our triangle into six little triangles like this: each small triangle is also a right triangle! and notice that 1 2=3, 1 3=4 and 2 3=5, making the 3,4,5 triangle. so.
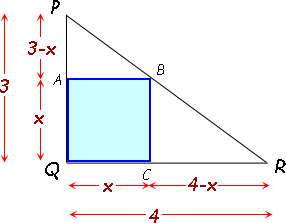
Inscribed Square In A Triangle Math Central Solution 2. the hypotenuse of the triangle is a diameter of the circumcircle, so it has length . the triangle is similar to a 3 4 5 triangle with the ratio of their side lengths equal to . the area of a 3 4 5 triangle is . the square of the ratio of their side lengths is equal to the ratio of their areas. call the area of the triangle . The 3,4,5 triangle has a simple beauty. at its heart is a circle: the circle is a unit circle: it has a radius of exactly 1 and an area of π. and using that circle we can cut our triangle into six little triangles like this: each small triangle is also a right triangle! and notice that 1 2=3, 1 3=4 and 2 3=5, making the 3,4,5 triangle. so.
How To Find Area Involving Inscribed Figures Geometry Study

Comments are closed.