Hyperbolic Functions Introduction

Introduction To Hyperbolic Functions Mr Mathematics Hyperbolic functions occur in the calculations of angles and distances in hyperbolic geometry. they also occur in the solutions of many linear differential equations (such as the equation defining a catenary), cubic equations, and laplace's equation in cartesian coordinates. laplace's equations are important in many areas of physics, including. The derivatives of hyperbolic functions are: d dx sinh (x) = cosh x; d dx cosh (x) = sinh x; some relations of hyperbolic function to the trigonometric function are as follows: sinh x = – i sin(ix) cosh x = cos (ix) tanh x = i tan(ix) hyperbolic function identities. the hyperbolic function identities are similar to the trigonometric functions.

Introduction To Hyperbolic Functions Youtube Hyperbolic functions are similar to trigonometric functions, but instead of unit circles, they are defined using rectangular hyperbolas. in trigonometry, the coordinates on a unit circle are represented as (cos θ, sin θ), whereas in hyperbolic functions, the pair (cosh θ, sinh θ) represents points on the right half of an equilateral hyperbola. Hyperbolas come from inversions (x y = 1 or y = 1 x). the area under an inversion grows logarithmically, and the corresponding coordinates grow exponentially. if we rotate the hyperbola, we rotate the formula to (x − y) (x y) = x 2 − y 2 = 1. the area coordinates now follow modified logarithms exponentials: the hyperbolic functions. Answer: hence we proved that cosh x sinh x = e x. example 3: prove the hyperbolic trig identity coth 2 x csch 2 x = 1. solution: to prove the identity coth 2 x csch 2 x = 1, we will use the following hyperbolic functions formulas: coth x = cosh x sinh x. csch x = 1 sinh x. consider lhs = coth 2 x csch 2 x. One of the interesting uses of hyperbolic functions is the curve made by suspended cables or chains. a hanging cable forms a curve called a catenary defined using the cosh function: f(x) = a cosh(x a) like in this example from the page arc length: other hyperbolic functions. from sinh and cosh we can create: hyperbolic tangent "tanh.

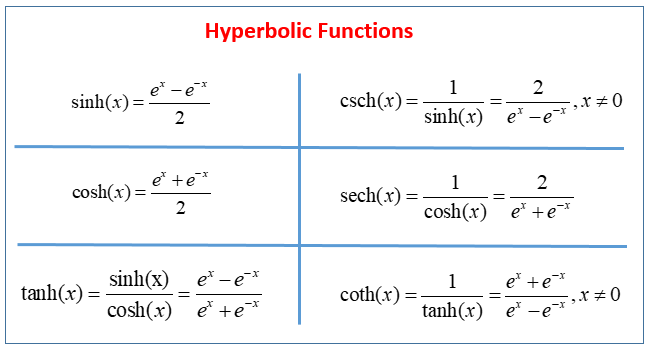
Hyperbolic Functions Solutions Examples Videos Answer: hence we proved that cosh x sinh x = e x. example 3: prove the hyperbolic trig identity coth 2 x csch 2 x = 1. solution: to prove the identity coth 2 x csch 2 x = 1, we will use the following hyperbolic functions formulas: coth x = cosh x sinh x. csch x = 1 sinh x. consider lhs = coth 2 x csch 2 x. One of the interesting uses of hyperbolic functions is the curve made by suspended cables or chains. a hanging cable forms a curve called a catenary defined using the cosh function: f(x) = a cosh(x a) like in this example from the page arc length: other hyperbolic functions. from sinh and cosh we can create: hyperbolic tangent "tanh. 1. introduction. in this video we shall define the three hyperbolic functions f(x) = sinh x, f(x) = cosh x and f(x) = tanh x. we shall look at the graphs of these functions, and investigate some of their properties. 2. defining f (x) = cosh x. the hyperbolic functions cosh x and sinh x are defined using the exponential function ex. Sinh. the six well‐known hyperbolic functions are the hyperbolic sine , hyperbolic cosine , hyperbolic tangent , hyperbolic cotangent , hyperbolic cosecant , and hyperbolic secant . they are among the most used elementary functions. the hyperbolic functions share many common properties and they have many properties and formulas that are.

Complex Numbers Hyperbolic Functions Introduction And Formulae Youtube 1. introduction. in this video we shall define the three hyperbolic functions f(x) = sinh x, f(x) = cosh x and f(x) = tanh x. we shall look at the graphs of these functions, and investigate some of their properties. 2. defining f (x) = cosh x. the hyperbolic functions cosh x and sinh x are defined using the exponential function ex. Sinh. the six well‐known hyperbolic functions are the hyperbolic sine , hyperbolic cosine , hyperbolic tangent , hyperbolic cotangent , hyperbolic cosecant , and hyperbolic secant . they are among the most used elementary functions. the hyperbolic functions share many common properties and they have many properties and formulas that are.