Ii Compound Pendulum In Hamiltonian Formulation Ii With Notes ођ

Ii Compound Pendulum In Hamiltonian Formulation Ii With Notes Youtube Hello everyone **i am nagarjun sahu & you are watching my you tube channel arjun physics classes °°°in this channel you will get easiest explanati. 2. where, i is the moment of inertia of the body through the axis p. expression (2) represents a simple harmonic motion and hence the time period of oscillation is given by. = 2 √ (3) now, i = ig ma2, where ig is moment of inertia of the body about an axis parallel with axis of oscillation and passing through the center of gravity g.

Ii Simple Pendulum In Hamiltonian Formulation Ii With Notes Youtube Which, in this case, coincides with what we usually call momentum. the hamiltonian is then given by h = p·r˙ l = 1 2m p2 v(r)(4.19) where, in the end, we’ve eliminated r˙ in favour of p and written the hamiltonian as a function of p and r. hamilton’s equations are simply r˙ = @h @p = 1 m p p˙ = @h @r = rv (4.20). The hamiltonian formulation. the newtonian formulation involves the application of newton’s second law with: in the special case of a conservative system, the forces acting on the particles are derived here from a scalar potential energy function this is the approach taken in molecular dynamics simulations. newtonian formulation. This derivation follows the hamiltonian formulation of the dynamics of the double compound pendulum and is slightly more general than that given in this page. the pendulum rods are of lengths l1 l 1 and l2 l 2 and have masses m1 m 1 and m2 m 2 uniformly distributed along their lengths. the coordinate system used is illustrated below. Finally, if =ˇ=2, i.e., the pendulum is sticking out to the right, then lcos( )= lcos(ˇ=2)= l(0)=0, i.e., the endpoint is also sitting right along the ground. perfect. so, we can write our gravitational potential energy as u =mgh= mglcos( ). this line of thinking will also come in handy when we consider a general compound pendulum. 2.

Hamiltonian For Compund Pendulum And Derivation Of Equation Of Motion This derivation follows the hamiltonian formulation of the dynamics of the double compound pendulum and is slightly more general than that given in this page. the pendulum rods are of lengths l1 l 1 and l2 l 2 and have masses m1 m 1 and m2 m 2 uniformly distributed along their lengths. the coordinate system used is illustrated below. Finally, if =ˇ=2, i.e., the pendulum is sticking out to the right, then lcos( )= lcos(ˇ=2)= l(0)=0, i.e., the endpoint is also sitting right along the ground. perfect. so, we can write our gravitational potential energy as u =mgh= mglcos( ). this line of thinking will also come in handy when we consider a general compound pendulum. 2. This lecture speaks about the derivation of the equation of motion of compound pendulum using hamilton's equation. the lecture starts with the introduction o. Exercises in classical mechanics. hamiltonian formalism for the double pendulum(10 points) consider a double pendulum that consists of two massless rods of length l1 and. 2 with masses m1 and m2 attached to their ends. the ̄rst pendulum is attached. o a ̄xed point and can freely swing about it. the second pendulum is attached to the.
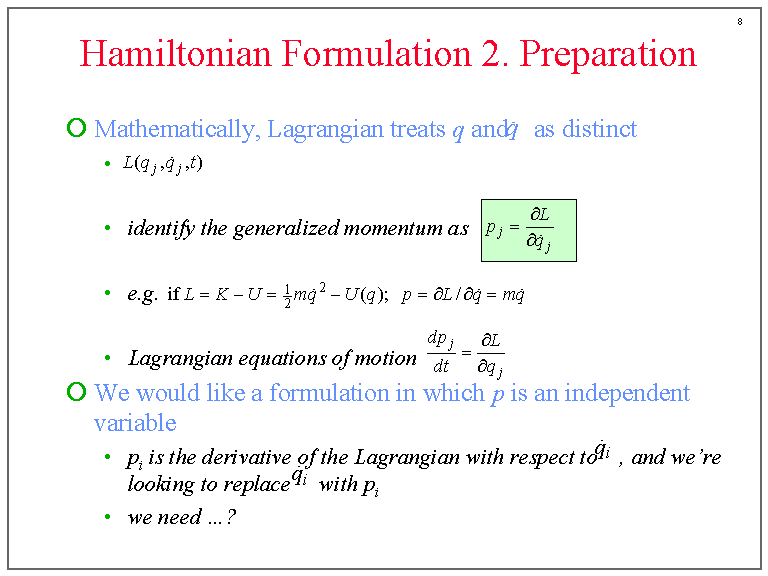
Hamiltonian Formulation 2 Preparation This lecture speaks about the derivation of the equation of motion of compound pendulum using hamilton's equation. the lecture starts with the introduction o. Exercises in classical mechanics. hamiltonian formalism for the double pendulum(10 points) consider a double pendulum that consists of two massless rods of length l1 and. 2 with masses m1 and m2 attached to their ends. the ̄rst pendulum is attached. o a ̄xed point and can freely swing about it. the second pendulum is attached to the.

Compound Pendulum Hamiltonian Formulation Equation Of Motion Of

Comments are closed.