Midpoint Theorem And Converse Of Midpoint Theorem In Triangle
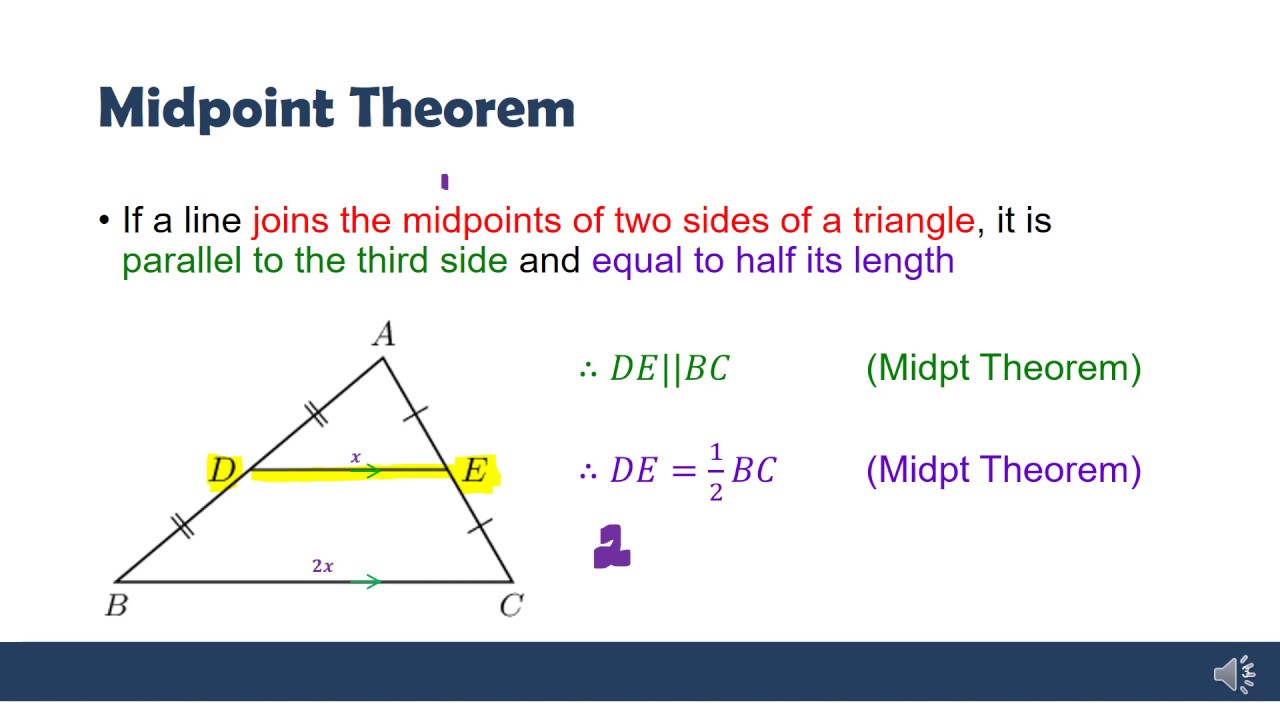
Gr 10 Geometry Triangles Midpoint Theorem And Converse Part 1 2 The midpoint theorem converse states that the line drawn through the midpoint of one side of a triangle that is parallel to another side will bisect the third side. midpoint theorem examples example 1: consider a triangle abc, and let d be any point on bc. The example is given below to understand the midpoint theorem. example: in triangle abc, the midpoints of bc, ca, and ab are d, e, and f, respectively. find the value of ef, if the value of bc = 14 cm. solution: given: bc = 14 cm. if f is the midpoint of ab and e is the midpoint of ac, then using the midpoint theorem:.

Midpoint Theorem Proof Formula Examples And Diagrams By converse of midpoint theorem, we know that the line is drawn from the midpoint of one side parallel to the other side and bisects the third side of the triangle. thus the line d o bisects the third line a x. a o = 1 2 a x = 1 2 (9 c m) = 4.5 c m. hence, the value of a o is 4.5 c m. q.4. The midpoint theorem generalizes to the intercept theorem, where rather than using midpoints, both sides are partitioned in the same ratio. [1] [2] the converse of the theorem is true as well. that is if a line is drawn through the midpoint of triangle side parallel to another triangle side then the line will bisect the third side of the triangle. Hence, the midpoint theorem is proved by (vi) and (x). converse of mid point theorem. according to the converse of the mid point theorem, if a line drawn through the midpoint of one side of a triangle is parallel to another side, it will bisect the third side. let abc be a triangle where d is the midpoint of ab. So, de:ef = 1:1 (as they are equal) example 2: in the figure given below l, m and n are mid points of side pq, qr, and pr respectively of triangle pqr. if pq = 8cm, qr = 9cm and pr = 6cm. find the perimeter of the triangle formed by joining l, m, and n. solution: as l and n are mid points. by mid point theorem.

Gr 10 Geometry Triangles Midpoint Theorem And Converse Part 2 2 Hence, the midpoint theorem is proved by (vi) and (x). converse of mid point theorem. according to the converse of the mid point theorem, if a line drawn through the midpoint of one side of a triangle is parallel to another side, it will bisect the third side. let abc be a triangle where d is the midpoint of ab. So, de:ef = 1:1 (as they are equal) example 2: in the figure given below l, m and n are mid points of side pq, qr, and pr respectively of triangle pqr. if pq = 8cm, qr = 9cm and pr = 6cm. find the perimeter of the triangle formed by joining l, m, and n. solution: as l and n are mid points. by mid point theorem. The midpoint theorem. the midpoint theorem tells us that the line segment joining two sides of any triangle at their midpoints is parallel to the third side, and the line segment is half the length of that third side. this at first sounds like nothing but brave talk, so let's test it. the theorem has two assertions. The midpoint theorem is a theorem that states that the line segment formed by the two midpoints of the triangles’ two sides will have a length equal to half of the third side parallel to it. to better understand what the theorem states, take a look at the triangle $\delta abc$ shown below.

Midpoint Theorem Statement Proof Converse Examples The midpoint theorem. the midpoint theorem tells us that the line segment joining two sides of any triangle at their midpoints is parallel to the third side, and the line segment is half the length of that third side. this at first sounds like nothing but brave talk, so let's test it. the theorem has two assertions. The midpoint theorem is a theorem that states that the line segment formed by the two midpoints of the triangles’ two sides will have a length equal to half of the third side parallel to it. to better understand what the theorem states, take a look at the triangle $\delta abc$ shown below.

Midpoint Theorem And Converse Of Midpoint Theorem In Triangle

Comments are closed.