Multiplication Addition Rule Probability Mutually Exclusive

Multiplication Addition Rule Probability Mutually Exclusive The addition rule. if a and b are defined on a sample space, then: p (a or b) = p (a) p (b) − p (a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.3.2 becomes. p (a or b) = p (a) p (b). example 4.3.1. klaus is trying to choose where to go on vacation. Example 8.2.5. if a card is drawn from a deck, use the addition rule to find the probability of obtaining an ace or a heart. solution. let a be the event that the card is an ace, and h the event that it is a heart. since there are four aces, and thirteen hearts in the deck, p (a) = 4 52 and p (h) = 13 52.
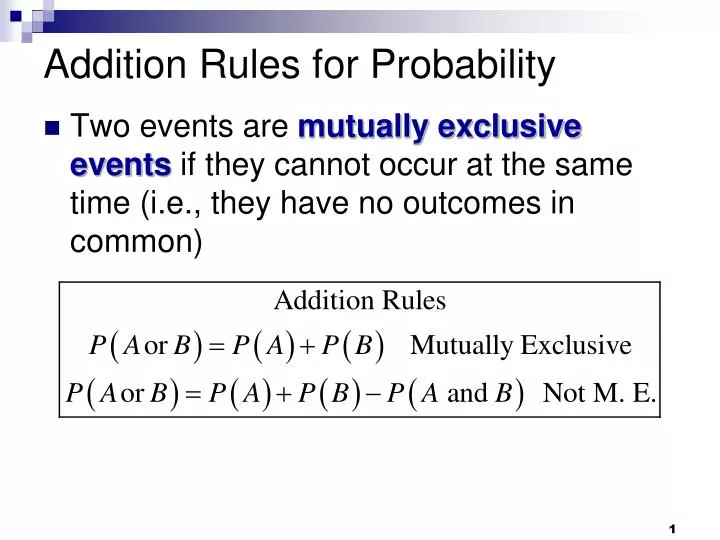
Ppt Addition Rules For Probability Powerpoint Presentation Free P(eorf) = p(e) p(f) − p(eand f) . it’s worth noting that this formula is truly an extension of the addition rule. remember that the addition rule requires that the events e and f are mutually exclusive. in that case, the compound event (e and f) is impossible, and so p(e and f) = 0. This video tutorial discusses the multiplication rule and addition rule of probability. it also explains how to determine if two events are independent even. Step 9: a helpful feature of tree diagrams is that the final outcomes are always mutually exclusive, so the addition rule can be directly applied. for example, the probability of drawing one suspect and one room (in any order) would be p ( s r ) p ( r s ) = 9 70 9 70 = 9 35 p ( s r ) p ( r s ) = 9 70 9 70 = 9 35 . When calculating probability, there are two rules to consider when determining if two events are independent or dependent and if they are mutually exclusive or not. the multiplication rule. if [latex]a[ latex] and [latex]b[ latex] are two events defined on a sample space, then: [latex]p(a \text{ and } b) = p(b)p(a|b)[ latex].
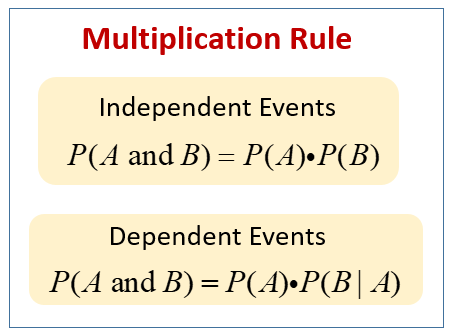
Multiplication Rule For Probability Examples Solutions Lessons Step 9: a helpful feature of tree diagrams is that the final outcomes are always mutually exclusive, so the addition rule can be directly applied. for example, the probability of drawing one suspect and one room (in any order) would be p ( s r ) p ( r s ) = 9 70 9 70 = 9 35 p ( s r ) p ( r s ) = 9 70 9 70 = 9 35 . When calculating probability, there are two rules to consider when determining if two events are independent or dependent and if they are mutually exclusive or not. the multiplication rule. if [latex]a[ latex] and [latex]b[ latex] are two events defined on a sample space, then: [latex]p(a \text{ and } b) = p(b)p(a|b)[ latex]. The addition rule. if a and b are defined on a sample space, then: p(a or b) = p(a) p(b) − p(a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.2.2 becomes. p(a or b) = p(a) p(b). example 4.2.1. klaus is trying to choose where to go on vacation. 15 3. 3 2 1. and, p (1st was a 10 Ω resistor and 2nd was a 30 Ω resistor) = =. 8 × 3 4 (c) as there are ten 30 Ω resistors in the box that contains a total of 6 10 = 16 resistors, and there is an equally likely chance of any resistor being selected, then. 10 5. p (1st selected is a 30 Ω resistor) =.

Probability Addition And Multiplication Rule Mutually Exclusive And The addition rule. if a and b are defined on a sample space, then: p(a or b) = p(a) p(b) − p(a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.2.2 becomes. p(a or b) = p(a) p(b). example 4.2.1. klaus is trying to choose where to go on vacation. 15 3. 3 2 1. and, p (1st was a 10 Ω resistor and 2nd was a 30 Ω resistor) = =. 8 × 3 4 (c) as there are ten 30 Ω resistors in the box that contains a total of 6 10 = 16 resistors, and there is an equally likely chance of any resistor being selected, then. 10 5. p (1st selected is a 30 Ω resistor) =.

Comments are closed.