Multiplication Addition Rule Probability Mutually Exclusive The addition rule. if a and b are defined on a sample space, then: p (a or b) = p (a) p (b) − p (a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.3.2 becomes. p (a or b) = p (a) p (b). example 4.3.1. klaus is trying to choose where to go on vacation. This video tutorial discusses the multiplication rule and addition rule of probability. it also explains how to determine if two events are independent even.
Probability Addition And Multiplication Rule Mutually Exclusive And P(eorf) = p(e) p(f) − p(eand f) . it’s worth noting that this formula is truly an extension of the addition rule. remember that the addition rule requires that the events e and f are mutually exclusive. in that case, the compound event (e and f) is impossible, and so p(e and f) = 0. Chapter 3 probability: events and probabilities complement rule: for any event a: p(a) p(a ) = 1 p(a ) = 1 p(a) two events are mutually exclusive if they can not both happen: p(a and b) = 0 to check if two events a, b are mutually exclusive, find p(a and b) and see if it is equal to 0. example 3: two coins are tossed. Mutually exclusive events: two events are mutually exclusive if they can not both happen. (we called this disjoint with sets). p(e∩ f)= p(e and f)=0 when rolling two dice, the events of “getting a sum of 7”, “getting a double” are mutually exclusive because they can not both happen when rolling two dice. From the addition rule above, we can conclude that the subtraction rule for mutually exclusive events takes the form; conditional probability for mutually exclusive events. we have defined conditional probability with the following equation: we can redefine the above using the multiplication rule. hence. below is a venn diagram of a set.
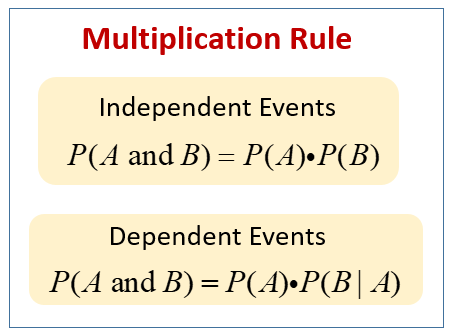
Multiplication Rule For Probability Examples Solutions Lessons Mutually exclusive events: two events are mutually exclusive if they can not both happen. (we called this disjoint with sets). p(e∩ f)= p(e and f)=0 when rolling two dice, the events of “getting a sum of 7”, “getting a double” are mutually exclusive because they can not both happen when rolling two dice. From the addition rule above, we can conclude that the subtraction rule for mutually exclusive events takes the form; conditional probability for mutually exclusive events. we have defined conditional probability with the following equation: we can redefine the above using the multiplication rule. hence. below is a venn diagram of a set. Addition rule formula. when calculating the probability of either one of two events from occurring, it is as simple as adding the probability of each event and then subtracting the probability of both of the events occurring: p (a or b) = p (a) p (b) p (a and b) we must subtract p (a and b) to avoid double counting!. The addition rule. if a and b are defined on a sample space, then: p(a or b) = p(a) p(b) − p(a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.2.2 becomes. p(a or b) = p(a) p(b). example 4.2.1. klaus is trying to choose where to go on vacation.
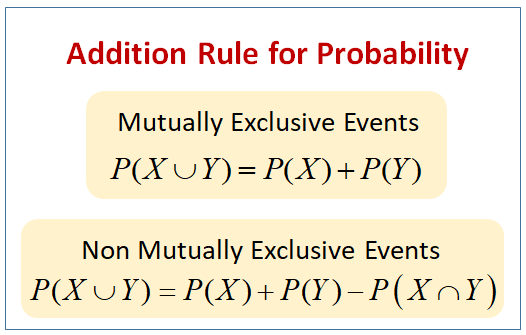
Bayes Theorem Solutions Formulas Examples Videos Addition rule formula. when calculating the probability of either one of two events from occurring, it is as simple as adding the probability of each event and then subtracting the probability of both of the events occurring: p (a or b) = p (a) p (b) p (a and b) we must subtract p (a and b) to avoid double counting!. The addition rule. if a and b are defined on a sample space, then: p(a or b) = p(a) p(b) − p(a and b) if a and b are mutually exclusive, then. p(a and b) = 0. and equation 4.2.2 becomes. p(a or b) = p(a) p(b). example 4.2.1. klaus is trying to choose where to go on vacation.
Comments are closed.