Problem Solving With Gsp Three Tangent Circles Soddy Circles Youtube

Problem Solving With Gsp Three Tangent Circles Soddy Circles Youtube แสดงการใช้โปรแกรม the geometer's sketchpad program ในการสร้างรูปวงกลมสัมผัสกัน. Learn to apply systems of equations in solving geometry problems. how to find the radii of three circles touching each other externally or externally tangent.

Construction Of Sangaku Using Gsp 3 Inscribed Tangent Circle Youtube A special case of apollonius' problem requiring the determination of a circle touching three mutually tangent circles (also called the kissing circles problem). there are two solutions: a small circle surrounded by the three original circles, and a large circle surrounding the original three. frederick soddy gave the formula for finding the radius of the so called inner and outer soddy circles. 0. if the radius of the circle is 1, the radius of three circles that will be internally tangent is 2 3–√ − 3 ≈ 0.464 2 3 − 3 ≈ 0.464 by soddy's formula, given in the soddy's circles section of this. you can construct this length, then mark it off on a diameter of the circle to find one of the centers, and finish constructing the. Consider three mutually tangent circles, and draw their inner soddy circle. then draw the inner soddy circles of this circle with each pair of the original three, and continue iteratively. the steps in the process are illustrated above (trott 2004, pp. 34 35). an animation illustrating the construction of the gasket is shown above. the points which are never inside a circle form a set of. Given three noncollinear points, construct three tangent circles such that one is centered at each point and the circles are pairwise tangent to one another. then there exist exactly two nonintersecting circles that are tangent to all three circles. these are called the inner and outer soddy circles, and their centers are called the inner s and outer soddy centers s^', respectively. frederick.

Problem Solving With Gsp Set Of Circles Tangent To Two Given Circles Consider three mutually tangent circles, and draw their inner soddy circle. then draw the inner soddy circles of this circle with each pair of the original three, and continue iteratively. the steps in the process are illustrated above (trott 2004, pp. 34 35). an animation illustrating the construction of the gasket is shown above. the points which are never inside a circle form a set of. Given three noncollinear points, construct three tangent circles such that one is centered at each point and the circles are pairwise tangent to one another. then there exist exactly two nonintersecting circles that are tangent to all three circles. these are called the inner and outer soddy circles, and their centers are called the inner s and outer soddy centers s^', respectively. frederick. We have now constructed the three tangent circles. tangent circle gsp sketch. but how are the other two tangent circles constructed? these circles are a little more complicated. let’s first construct the outer soddy circle: outer soddy circle: given triangle abc, step 1: with ab as the smallest side, construct a point x on side ac so that ax. The circles are correspondingly called the outer and the inner soddy circles. their centers are also known as the soddy points of the triangle formed by the centers of the triplet. (this is a particular case of the more general apollonius' problem that asks for a circle tangent to the given three circles (or lines, or passing through given.
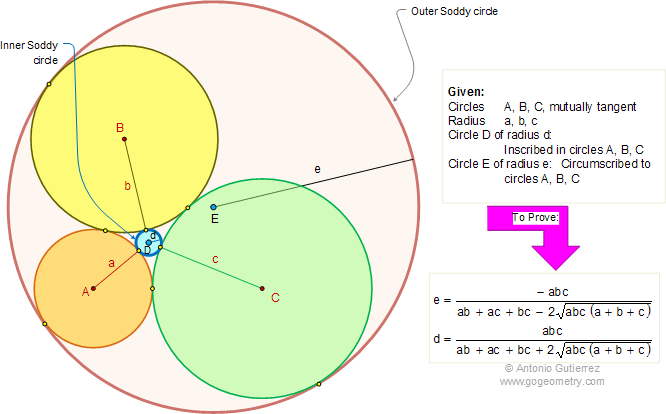
Soddy Circles And Descartes Theorem Three Tangent Circles Ipad Apps We have now constructed the three tangent circles. tangent circle gsp sketch. but how are the other two tangent circles constructed? these circles are a little more complicated. let’s first construct the outer soddy circle: outer soddy circle: given triangle abc, step 1: with ab as the smallest side, construct a point x on side ac so that ax. The circles are correspondingly called the outer and the inner soddy circles. their centers are also known as the soddy points of the triangle formed by the centers of the triplet. (this is a particular case of the more general apollonius' problem that asks for a circle tangent to the given three circles (or lines, or passing through given.

910 Ge Three Circles Tangent To Each Other Youtube

Comments are closed.