Prove Trigonometric Identities Part 1

Prove Trigonometric Identities Part 1 Youtube Free trigonometric identity calculator verify trigonometric identities step by step trigonometric identity proving calculator. en. related symbolab blog posts. This lesson shows four examples (3 in part 1) regarding how to prove trigonometric identities. this is the first part of a two part lesson. this lesson was.

Proofs With Trigonometric Identities Part 1 Youtube There are multiple ways to represent a trigonometric expression. verifying the identities illustrates how expressions can be rewritten to simplify a problem. graphing both sides of an identity will verify it. simplifying one side of the equation to equal the other side is another method for verifying an identity. Consequently, any trigonometric identity can be written in many ways. to verify the trigonometric identities, we usually start with the more complicated side of the equation and essentially rewrite the expression until it has been transformed into the same expression as the other side of the equation. Proving trigonometric identities basic. trigonometric identities are equalities involving trigonometric functions. an example of a trigonometric identity is. \sin^2 \theta \cos^2 \theta = 1. sin2 θ cos2 θ = 1. in order to prove trigonometric identities, we generally use other known identities such as pythagorean identities. The trigonometric identities are equations that are true for right angled triangles. periodicity of trig functions. sine, cosine, secant, and cosecant have period 2π while tangent and cotangent have period π. identities for negative angles. sine, tangent, cotangent, and cosecant are odd functions while cosine and secant are even functions.

How To Prove Trigonometric Identities Trigonometry Study Proving trigonometric identities basic. trigonometric identities are equalities involving trigonometric functions. an example of a trigonometric identity is. \sin^2 \theta \cos^2 \theta = 1. sin2 θ cos2 θ = 1. in order to prove trigonometric identities, we generally use other known identities such as pythagorean identities. The trigonometric identities are equations that are true for right angled triangles. periodicity of trig functions. sine, cosine, secant, and cosecant have period 2π while tangent and cotangent have period π. identities for negative angles. sine, tangent, cotangent, and cosecant are odd functions while cosine and secant are even functions. 1 tan2θ = 1 (sinθ cosθ)2 rewrite left side = (cosθ cosθ)2 (sinθ cosθ)2 write both terms with the common denominator = cos2θ sin2θ cos2θ = 1 cos2θ = sec2θ. recall that we determined which trigonometric functions are odd and which are even. the next set of fundamental identities is the set of even odd identities. Introduction to trigonometric identities and equations; 7.1 simplifying and verifying trigonometric identities; 7.2 sum and difference identities; 7.3 double angle, half angle, and reduction formulas; 7.4 sum to product and product to sum formulas; 7.5 solving trigonometric equations; 7.6 modeling with trigonometric functions.

Math Rescue Trigonometry Proving Trigonometric Identities 1 tan2θ = 1 (sinθ cosθ)2 rewrite left side = (cosθ cosθ)2 (sinθ cosθ)2 write both terms with the common denominator = cos2θ sin2θ cos2θ = 1 cos2θ = sec2θ. recall that we determined which trigonometric functions are odd and which are even. the next set of fundamental identities is the set of even odd identities. Introduction to trigonometric identities and equations; 7.1 simplifying and verifying trigonometric identities; 7.2 sum and difference identities; 7.3 double angle, half angle, and reduction formulas; 7.4 sum to product and product to sum formulas; 7.5 solving trigonometric equations; 7.6 modeling with trigonometric functions.

Prove Trigonometry Identities Double Angle Identities Part 1 Osman

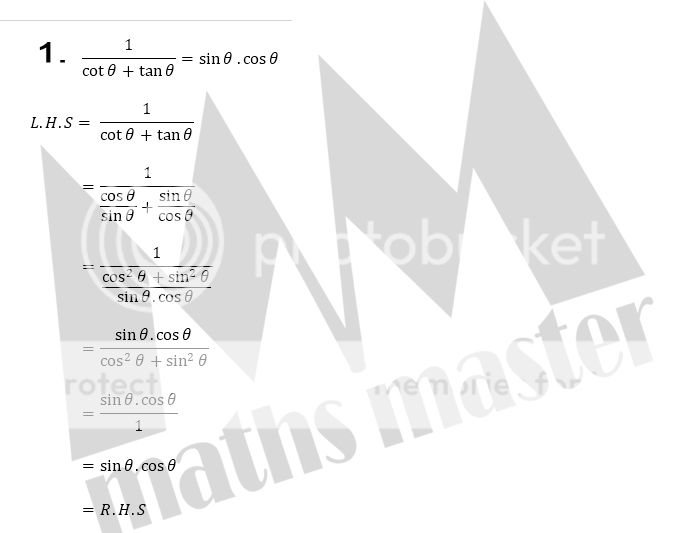
Trigonometric Identities Exercises Part 1 Maths Master