Solved 1 5 Points Total Consider The Second Order Linear Chegg

Solved 1 5 Points Total Consider The Second Order Linear Chegg Question: 1. (5 points total) consider the second order linear equation a (x)y ′′ b (x)y ′ c (x)y = 0 (1) let y1 and y2 be two solutions to this differential equation on an open interval i where a,b,c are continuous and a (x) is never zero. a. (1 point) let w (y1, y2) =w (x). by writing out the wronskian and taking the derivative, show. Step 1. (5 points total) consider the second order linear equation a(x)y′′ b(x)y′ c (x)y =0 let y1 and y2 be two solutions to this differential equation on an open interval i where a,b,c are continuous and a(x) is never zero. a. (1 point) let w (y1,y2)=w (x). by writing out the wronskian and taking the derivative, show that a(x)dxdw =(y1.

Solved 1 5 Points Total Consider The Linear Chegg Describe how to reduce the order of the original problem, by setting up a second order linear homogeneous differential equation to solve for u. specifically, find this second order differential equation (you do not need to solve it). 4. (5 points) consider the following linear constant coefficient homogeneous differential equation dy do 0. An important difference between first order and second order equations is that, with second order equations, we typically need to find two different solutions to the equation to find the general solution. if we find two solutions, then any linear combination of these solutions is also a solution. we state this fact as the following theorem. Definition: homogeneous and nonhomogeneous linear equations. a second order differential equation is linear if it can be written in the form. a2(x)y ″ a)1(x)y ′ a0(x)y = r(x), where a2(x), a1(x), a0(x), and r(x) are real valued functions and a2(x) is not identically zero. if r(x) ≡ 0 —in other words, if r(x) = 0 for every value of x. 4 classification of second order equations. 4.1 types of second order equations. ond order equationsf (~x; u; du; d2u) = 0:in general, higher order equations are more comp. icated to solve than first order equations. consequently. we will only be studying linear equations. first, let’s consider a second order.
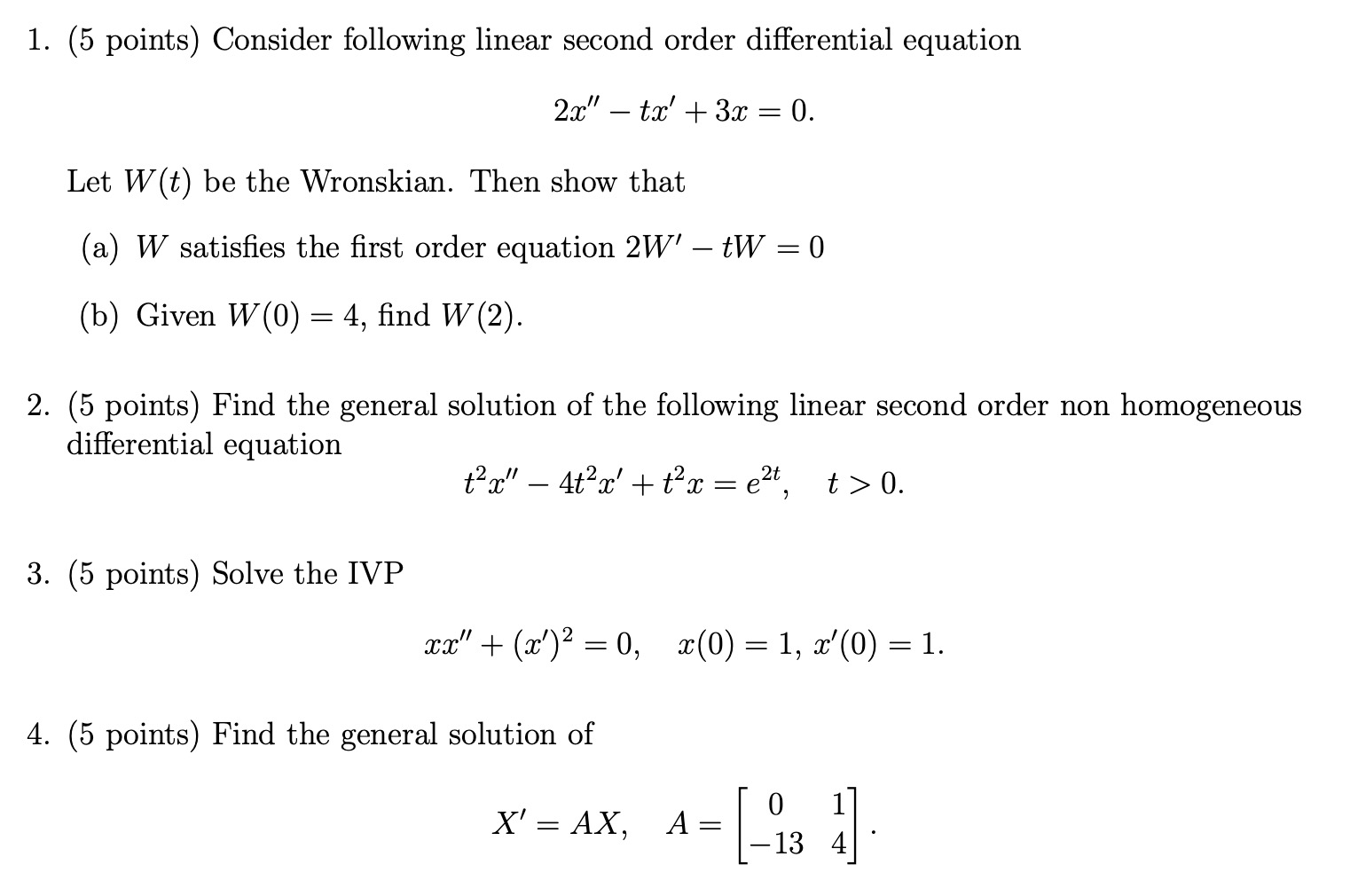
Solved 1 5 Points Consider Following Linear Second Order Chegg Definition: homogeneous and nonhomogeneous linear equations. a second order differential equation is linear if it can be written in the form. a2(x)y ″ a)1(x)y ′ a0(x)y = r(x), where a2(x), a1(x), a0(x), and r(x) are real valued functions and a2(x) is not identically zero. if r(x) ≡ 0 —in other words, if r(x) = 0 for every value of x. 4 classification of second order equations. 4.1 types of second order equations. ond order equationsf (~x; u; du; d2u) = 0:in general, higher order equations are more comp. icated to solve than first order equations. consequently. we will only be studying linear equations. first, let’s consider a second order. K. k k is described by the simple harmonic oscillation, whose equation of motion is given in the form of a homogeneous second order linear differential equation: m d 2 x d t 2 k x = 0. m \frac {\mathrm {d}^2x} {\mathrm {d}t^2} k x = 0 mdt2d2x kx = 0. adding the damping term in the equation of motion, the equation of motion is given as. 3 second order linear odes: context 3.1 a rst example before getting to the general theory, let’s explore the structure with an example. consider the second order linear ode (for y(t)) y00 y0 2y= 0 note that the operator here is ly= y00 y0 2y, and the ode is ly= 0. let’s search for solutions by the method of guessing. we know that ert is.
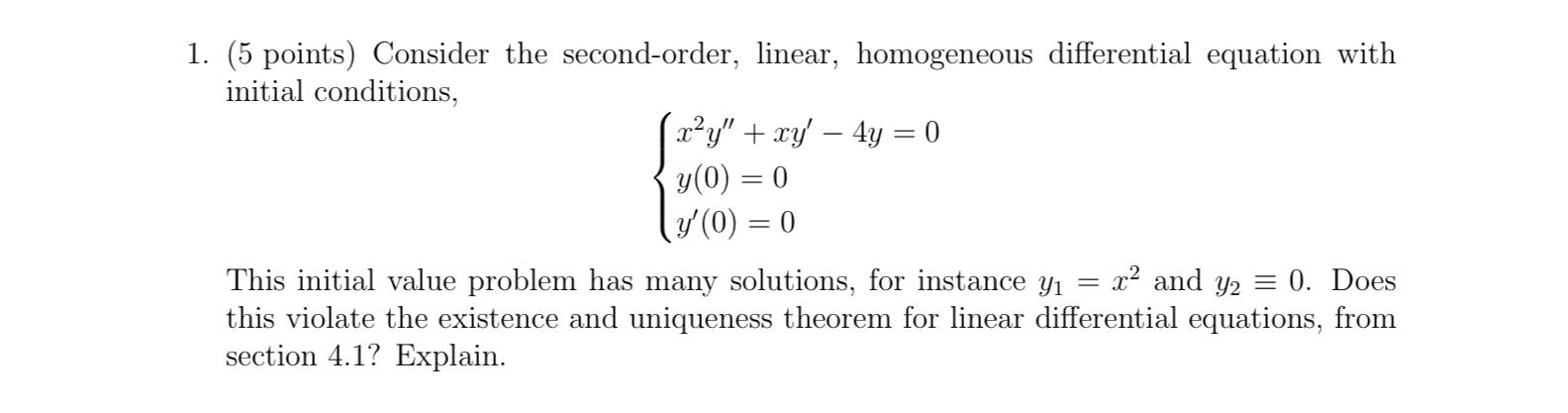
Solved 1 5 Points Consider The Second Order Linear Chegg K. k k is described by the simple harmonic oscillation, whose equation of motion is given in the form of a homogeneous second order linear differential equation: m d 2 x d t 2 k x = 0. m \frac {\mathrm {d}^2x} {\mathrm {d}t^2} k x = 0 mdt2d2x kx = 0. adding the damping term in the equation of motion, the equation of motion is given as. 3 second order linear odes: context 3.1 a rst example before getting to the general theory, let’s explore the structure with an example. consider the second order linear ode (for y(t)) y00 y0 2y= 0 note that the operator here is ly= y00 y0 2y, and the ode is ly= 0. let’s search for solutions by the method of guessing. we know that ert is.

Comments are closed.