Triangle Abc Is An Equilateral Ad Bisects Angle Bac Show That Triangle Abd о
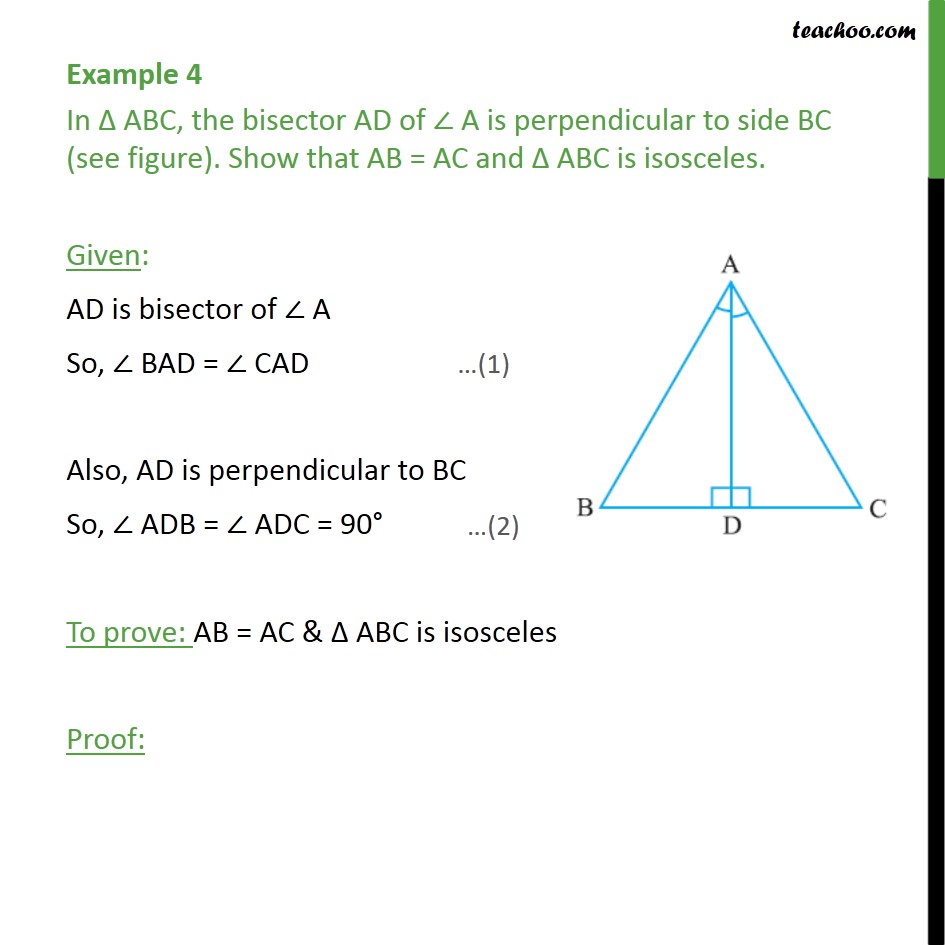
Example 4 In Triangle Abc The Bisector Ad Of Angle A Is Perpendicul In ∆abc, we are given that it is an equilateral triangle, so all three sides are congruent. let's denote the length of each side as 's'. now, since ad is the angle bisector of ∠bac, it divides it into two congruent angles, so m∠bad = m∠cad. since ∆abc is equilateral, all angles are congruent, so m∠bac = m∠bca = m∠cab. We can see that the small triangle fits into the big triangle four times. so when the lengths are twice as long, the area is four times as big. so the ratio of their areas is 4:1. we can also write 4:1 as 2 2:1. the general case: triangles abc and pqr are similar and have sides in the ratio x:y. we can find the areas using this formula from.
34 In Triangle Abc Angle Bisects Angle A And Angle C Angle B Prove The angle bisector theorem is concerned with the relative lengths of the two segments that a triangle's side is divided into by a line that bisects the opposite angle. it equates their relative lengths to the relative lengths of the other two sides of the triangle. to bisect an angle means to cut it into two equal parts or angles. say that we wanted to bisect a 50 degree angle, then we. Solution: by the angle bisector theorem, or . plugging this into and solving for ac gives . we can plug this back in to find . in triangle abc, let p be a point on bc and let . find the value of . solution: first, we notice that . thus, ap is the angle bisector of angle a, making our answer 0. part (b), 1959 imo problems problem 5. Angle fed = x; fe=fd; fd=eo; triangle efa is congruent to triangle edo; triangle efa is similar to triangle abd; now construct the line fo and drop a perpendicular from o to point g on bc. then the following can be proved: fo bisects angle eod into x x and also bisects angle fb into (180 3x) (180 3x) the points f o and g are colinear; bg = gc. The angle bisector is a line that divides an angle into two equal halves, each with the same angle measure. the angle bisector theorem states that in a triangle, the angle bisector partitions the opposite side of the triangle into two segments, with a ratio that is the same as the ratio between the two sides forming the angle it bisects: if ∠.

In Quadrilateral Abcd Ac Ad And Ab Bisects Angle A Show That Angle fed = x; fe=fd; fd=eo; triangle efa is congruent to triangle edo; triangle efa is similar to triangle abd; now construct the line fo and drop a perpendicular from o to point g on bc. then the following can be proved: fo bisects angle eod into x x and also bisects angle fb into (180 3x) (180 3x) the points f o and g are colinear; bg = gc. The angle bisector is a line that divides an angle into two equal halves, each with the same angle measure. the angle bisector theorem states that in a triangle, the angle bisector partitions the opposite side of the triangle into two segments, with a ratio that is the same as the ratio between the two sides forming the angle it bisects: if ∠. Note that the bisector and the external bisector are uniquely defined by the angle. exercise 8.4.1. show that for any angle, its bisector and external bisector are perpendicular. hint. the bisectors of ∠abc, ∠bca, and ∠cab of a nondegenerate triangle abc are called bisectors of the triangle abc at vertexes a, b, and c respectively. lemma. Show that(i) Δabe ≅ Δacf(ii) ab = ac, i.e., abc is an isosceles triangle. abc and dbc are two isosceles triangles on the same base bc (see fig. 7.33). show that ∠abd = ∠acd. Δabc is an isosceles triangle in which ab = ac. side ba is produced to d such that ad = ab (see fig. 7.34). show that ∠bcd is a right angle.
Abc Is A Triangle In Which Angle B 2angle C D Is A Point On Side Bc Note that the bisector and the external bisector are uniquely defined by the angle. exercise 8.4.1. show that for any angle, its bisector and external bisector are perpendicular. hint. the bisectors of ∠abc, ∠bca, and ∠cab of a nondegenerate triangle abc are called bisectors of the triangle abc at vertexes a, b, and c respectively. lemma. Show that(i) Δabe ≅ Δacf(ii) ab = ac, i.e., abc is an isosceles triangle. abc and dbc are two isosceles triangles on the same base bc (see fig. 7.33). show that ∠abd = ∠acd. Δabc is an isosceles triangle in which ab = ac. side ba is produced to d such that ad = ab (see fig. 7.34). show that ∠bcd is a right angle.

In Abc If Ad Is The Angle Bisector Of Bac And Abc Is An Isosceles
8 In Triangle Abc Ab Ac D Is The Mid Point In The Interior Of

Comments are closed.